Science:Math Exam Resources/Courses/MATH101/April 2018/Question 07 (ii)

MATH101 April 2018

  •  Q1 (i)  •  Q1 (ii)  •  Q1 (iii)  •  Q1 (iv)  •  Q2 (i)  •  Q2 (ii)  •  Q2 (iii)  •  Q3 (i)  •  Q3 (ii)  •  Q4 (i)  •  Q4 (ii)  •  Q5 (i)  •  Q5 (ii)  •  Q6 (i)  •  Q6 (ii)  •  Q7 (i)  •  Q7 (ii)  •  Q8 (i)  •  Q8 (ii)  •  Q9 (i)  •  Q9 (ii)  •  Q10 (i)  •  Q10 (ii)  •  Q10 (iii)  •  Q11 (i)  •  Q11 (ii)  •  

   Other MATH101 Exams

  •  April 2011  •  April 2010  •  April 2009  •  April 2008  •  April 2007  •  April 2005  •  April 2006  •  April 2012  •  April 2013  •  April 2014  •  April 2015  •  April 2016  •  April 2018  •  April 2017  •  

Question 07 (ii)

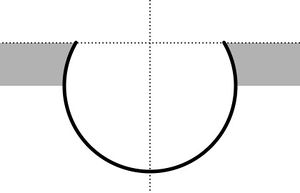
Let ${\textstyle {\mathcal {R}}}$ be the portion of the circle ${\textstyle (y+1)^{2}+x^{2}=4}$, with distances measured in metres, that lies to the right of the ${\textstyle y}$-axis and is below the horizontal line ${\textstyle y=0}$ denoting the ${\textstyle x}$-axis (see the plot below). A reservoir dug into the ground has the shape that results from the region ${\textstyle {\mathcal {R}}}$ being rotated around the ${\textstyle y}$-axis. A cross-section of the reservoir is shown below. (ii) The reservoir from part (i) is completely filled with a fluid of density ${\textstyle 1000\,{{\mbox{kg}}/{\mbox{m}}^{3}}}$. Find the work, in joules, required to pump all the fluid out of the top of the reservoir. Use ${\textstyle g=9.8}$ m${\textstyle {}/{}}$s${\textstyle {}^{2}}$ for the acceleration due to gravity. A calculator-ready answer is sufficient.

Make sure you understand the problem fully: What is the question asking you to do? Are there specific conditions or constraints that you should take note of? How will you know if your answer is correct from your work only? Can you rephrase the question in your own words in a way that makes sense to you?

If you are stuck, check the hints below. Read the first one and consider it for a while. Does it give you a new idea on how to approach the problem? If so, try it! If after a while you are still stuck, go for the next hint.

Hint 1
The work done by a force $F(y)$ in moving an object from $y=a$ to $y=b$ is $$W=\int _{a}^{b}F(y)dy.$$ (Ref. CLP–II INTEGRAL CALCULUS)

Hint 2
Use the Newton's second law of motion to find the force.

Hint 3
Recall that ${\text{(mass)}}={\text{(density)}}\cdot {\text{(volume)}}$.

Checking a solution serves two purposes: helping you if, after having used all the hints, you still are stuck on the problem; or if you have solved the problem and would like to check your work. If you are stuck on a problem: Read the solution slowly and as soon as you feel you could finish the problem on your own, hide it and work on the problem. Come back later to the solution if you are stuck or if you want to check your work. If you want to check your work: Don't only focus on the answer, problems are mostly marked for the work you do, make sure you understand all the steps that were required to complete the problem and see if you made mistakes or forgot some aspects. Your goal is to check that your mental process was correct, not only the result.

Solution

Found a typo? Is this solution unclear? Let us know here. Please rate my easiness! It's quick and helps everyone guide their studies. From the part (i), the volume of a cylindrical pancake at the height $y$ from $y=0$ is $$dV=\pi (4-(y+1)^{2})dy.$$ Combining with the constant density of the fluid ${\textstyle 1000\,({{\mbox{kg}}/{\mbox{m}}^{3}})}$, the mass of the pancake is $$dm=\rho dV=1000\pi (4-(y+1)^{2})dy.$$ Then, by Newton's second law of motion with ${\textstyle g=9.8({\mbox{m}}/{\mbox{s}}^{2})}$, we can raise the pancake by applying the compensating force to the pancake $$dF=gdm=(9.8)\cdot 1000\pi (4-(y+1)^{2})dy.$$ Finally, to move the fluid in the pancake at height $y$ out of the reservoir, we need to raise $-y({\mbox{m}})$, so that the required work is $$dW=(-y)dF=(9.8)\cdot 1000\pi (-y)(4-(y+1)^{2})dy.$$ Integrating it in the range $(-3,0)$, the work required to pump all the fluid out of the top of the reservoir is $${\begin{aligned}W&=\int _{-3}^{0}(9.8)\cdot 1000\pi (-y)(4-(y+1)^{2})dy=9800\pi \int _{-3}^{0}y^{3}+2y^{2}-3ydy\\&=9800\pi \left[{\frac {1}{4}}y^{4}+{\frac {2}{3}}y^{3}-{\frac {3}{2}}y^{2}\right]_{-3}^{0}=-9800\pi \left({\frac {1}{4}}(-3)^{4}+{\frac {2}{3}}(-3)^{3}-{\frac {3}{2}}(-3)^{2}\right)\\&=-9800\pi \left({\frac {81}{4}}-18-{\frac {27}{2}}\right)=9800\pi \cdot {\frac {45}{4}}=110250\pi .\end{aligned}}$$ Answer: $\color {blue}110250\pi$