Science:Math Exam Resources/Courses/MATH152/April 2011/Question A 14
• QA 1 • QA 2 • QA 3 • QA 4 • QA 5 • QA 6 • QA 7 • QA 8 • QA 9 • QA 10 • QA 11 • QA 12 • QA 13 • QA 14 • QA 15 • QA 16 • QA 17 • QA 18 • QA 19 • QA 20 • QA 21 • QA 22 • QA 23 • QA 24 • QA 25 • QA 26 • QA 27 • QA 28 • QA 29 • QA 30 • QB 1(a) • QB 1(b) • QB 1(c) • QB 2(a) • QB 2(b) • QB 3(a) • QB 3(b) • QB 3(c) • QB 4(a) • QB 4(b) • QB 4(c) • QB 4(d) • QB 5(a) • QB 5(b) • QB 5(c) • QB 6(a) • QB 6(b) • QB 6(c) • QB 6(d) • QB 6(e) •
[hide]Question A 14 |
|---|
|
The unit square in is the set of all points with 0 ≤ x ≤ 1 and 0 ≤ y ≤ 1. In questions A13 and A14 below, suppose each point in the unit square undergoes a linear transformation that has the given matrix representation . Draw a labelled sketch of the transformed points. |
|
Make sure you understand the problem fully: What is the question asking you to do? Are there specific conditions or constraints that you should take note of? How will you know if your answer is correct from your work only? Can you rephrase the question in your own words in a way that makes sense to you? |
|
If you are stuck, check the hint below. Consider it for a while. Does it give you a new idea on how to approach the problem? If so, try it! |
[show]Hint |
|---|
|
Checking a solution serves two purposes: helping you if, after having used the hint, you still are stuck on the problem; or if you have solved the problem and would like to check your work.
|
[show]Solution |
|---|



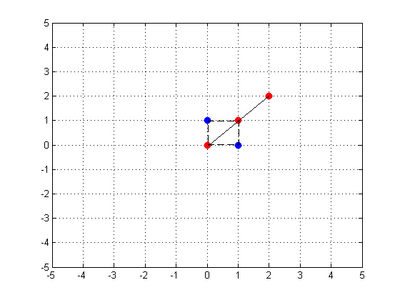
![{\displaystyle A=\left[{\begin{array}{cc}1&1\\1&1\end{array}}\right]}](https://wiki.ubc.ca/api/rest_v1/media/math/render/svg/f45117af5cbb0a07c431cf1da5e83fba768d2078)




![{\displaystyle {\color {blue}[0,0]}}](https://wiki.ubc.ca/api/rest_v1/media/math/render/svg/fb5b818bf367b1b69e5cc7453756efaf49c9f80a)
![{\displaystyle {\color {red}[0,0]}}](https://wiki.ubc.ca/api/rest_v1/media/math/render/svg/0c6a4c295888d65bf95e572157d600999723ee41)
![{\displaystyle {\color {blue}[1,0]}}](https://wiki.ubc.ca/api/rest_v1/media/math/render/svg/33bb3537600b670d5f14be2ce9cd31ce2fdbca48)
![{\displaystyle {\color {red}[1,1]}}](https://wiki.ubc.ca/api/rest_v1/media/math/render/svg/914e82e6f4c8e0ce787a5fed7eea84bc86e898d9)
![{\displaystyle {\color {blue}[0,1]}}](https://wiki.ubc.ca/api/rest_v1/media/math/render/svg/cd7c669fcb262fe0922b1d691ad619d4b79b954d)
![{\displaystyle {\color {blue}[1,1]}}](https://wiki.ubc.ca/api/rest_v1/media/math/render/svg/36078671931adef148f668b3404afa04f83ac630)
![{\displaystyle {\color {red}[2,2]}}](https://wiki.ubc.ca/api/rest_v1/media/math/render/svg/0c7a684c2c64e616d9eb9ccaf6c965f7073ff27c)