Found a typo? Is this solution unclear? Let us know here.
Please rate my easiness! It's quick and helps everyone guide their studies.
To determine the absolute max and min of the function f(x) on the interval we need to determine the critical points of the function and evaluate the function f(x) at those points, as well as the endpoints of the interval. The absolute max(min) of f(x) on the interval ![{\displaystyle [0,2\pi ]}](https://wiki.ubc.ca/api/rest_v1/media/math/render/svg/348d40bf3f8b7e1c00c4346440d7e2e4f0cc9b91) is given by the greatest(least) value of the function at those points. is given by the greatest(least) value of the function at those points.
To determine the critical points, we evaluate solve  for for  . .

and thus when 

If  is 0, then we know that is 0, then we know that  or or  . At these points, . At these points,  and thus and thus  . Hence we may suppose that . Hence we may suppose that  . .
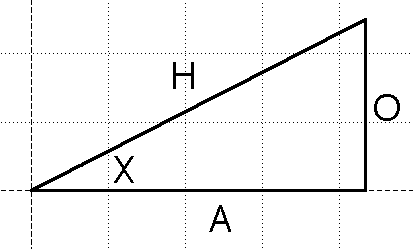
We know that  is negative in the second and fourth quadrants. We also know that a right triangle with side lengths is negative in the second and fourth quadrants. We also know that a right triangle with side lengths  has angles has angles  , ,  and and  . Thus, we have that . Thus, we have that  and and  (see the diagram below). (see the diagram below).
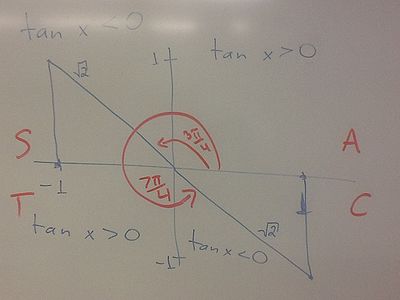
Thus, we plug in all these values to see that




and thus, the maximum occurs at  and its value is and its value is  and the minimum occurs at and the minimum occurs at  and its value is and its value is 
| 
![{\displaystyle [0,2\pi ].}](https://wiki.ubc.ca/api/rest_v1/media/math/render/svg/ff4354c1471c11d5701613ddb056258f5b7b8d19)

![{\displaystyle [0,2\pi ]}](https://wiki.ubc.ca/api/rest_v1/media/math/render/svg/348d40bf3f8b7e1c00c4346440d7e2e4f0cc9b91)