Science:Math Exam Resources/Courses/MATH101/April 2009/Question 07 (a)

MATH101 April 2009

• Q1 (a) • Q1 (b) • Q1 (c) • Q1 (d) • Q1 (e) • Q1 (f) • Q1 (g) • Q1 (h) • Q1 (i) • Q1 (j) • Q2 • Q3 (a) • Q3 (b) • Q4 (a) • Q4 (b) • Q5 • Q6 (a) • Q6 (b) • Q7 (a) • Q7 (b) • Q7 (c) • Q8 (a) • Q8 (b) •

Other MATH101 Exams

• April 2011 • April 2010 • April 2009 • April 2008 • April 2007 • April 2005 • April 2006 • April 2012 • April 2013 • April 2014 • April 2015 • April 2016 • April 2018 • April 2017 •

[hide] Question 07 (a)
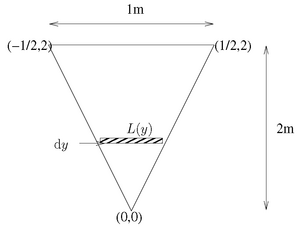
An open metal tank has two ends which are isosceles triangles with vertex at the bottom, two sides which are rectangular, and an open top. The tank is 1 metre wide, 2 metres deep, 10 metres long and full of water (density = 1000 kg/m³). (a) What is the hydrostatic force on each triangular end?

Make sure you understand the problem fully: What is the question asking you to do? Are there specific conditions or constraints that you should take note of? How will you know if your answer is correct from your work only? Can you rephrase the question in your own words in a way that makes sense to you?

If you are stuck, check the hints below. Read the first one and consider it for a while. Does it give you a new idea on how to approach the problem? If so, try it! If after a while you are still stuck, go for the next hint.

[show] Hint 1
Recall that hydrostatic pressure is given by, $\displaystyle {}P=\rho {g}h$ where $\rho$ is the density of water, g, the gravitational constant, and h the height from the top. It is important that height is measured from the top of the surface because as depth increases, there is more water above you, increasing to the pressure.

[show] Hint 2
Recall that they hydrostatic force on an object of area A at height h is $\displaystyle {}F=\rho {g}hA$ where $\rho$ is the density of the surrounding liquid.

Checking a solution serves two purposes: helping you if, after having used all the hints, you still are stuck on the problem; or if you have solved the problem and would like to check your work.

If you are stuck on a problem: Read the solution slowly and as soon as you feel you could finish the problem on your own, hide it and work on the problem. Come back later to the solution if you are stuck or if you want to check your work.
If you want to check your work: Don't only focus on the answer, problems are mostly marked for the work you do, make sure you understand all the steps that were required to complete the problem and see if you made mistakes or forgot some aspects. Your goal is to check that your mental process was correct, not only the result.

[show] Solution
Found a typo? Is this solution unclear? Let us know here. Please rate my easiness! It's quick and helps everyone guide their studies. Hydrostatic Force was not covered in the 2012 offering of this course. Time might better be spent solving other problems given how soon the exam is coming. Consider the triangular side pictured to the right . It has height 2 and length 1. Also consider a little rectangular sliver in the picture of length L(y) and height dy. The reason we consider rectangular pieces with constant height is because the pressure depends on height and so we would like the effect from height to be the same on any given rectangular piece. We will use the labelling in the diagram with the bottom vertex being (0,0), the right vertex being (1/2,2) and the left vertex being (-1/2,2). With our origin at the bottom, we will label y from there. This means that the height (as measured from the top of the surface) is (2-y). We then have that the force on any given rectangle, dF, is, $\displaystyle {}dF=\rho {g}(2-y)L(y){\textrm {d}}y.$ We could then get the total force by integrating dF but first we need an expression for the length in terms of the height, y. The length of any rectangle will start at the left edge of the triangle and end at the right edge. We can use the coordinates that we listed to find equations for these lines. The right edge is given by $\displaystyle y=4x$ which written in terms of y is, $x_{R}={\frac {y}{4}}$ where we have added a subscript R to indicate it's the right edge. Notice we compute the line by finding the slope using the right vertex and bottom vertex and then notice that the line goes through our origin to its y-intercept is zero. We can similarly get that the left edge is $x_{L}=-{\frac {y}{4}}.$ This can also be argued with symmetry of the right edge. We then have that $L(y)=x_{R}-x_{L}={\frac {y}{4}}-\left(-{\frac {y}{4}}\right)={\frac {y}{2}}.$ Therefore we can now get F via integration where we see that y starts at zero and ends at y=2. Therefore, $F=\int _{0}^{2}\rho {g}(2-y)L(y){\textrm {d}}y=\int _{0}^{2}\rho {g}{\frac {2y-y^{2}}{2}}{\textrm {d}}y=\rho {g}\left.\left({\frac {y^{2}}{2}}-{\frac {y^{3}}{6}}\right)\right\|_{0}^{2}={\frac {2\rho {g}}{3}}.$ We are told in the problem that the density is 1000kg/m^3 and we take the gravitational constant as 9.8N/kg. Therefore, $F={\frac {2}{3}}\cdot 9800={\frac {19600}{3}}N.$ This is the hydrostatic force on each of the triangular sides.