Science:Math Exam Resources/Courses/MATH101/April 2008/Question 02 (c)

MATH101 April 2008

  •  Q1 (a)  •  Q1 (b)  •  Q1 (c)  •  Q1 (d)  •  Q1 (e)  •  Q1 (f)  •  Q1 (g)  •  Q2 (a)  •  Q2 (b)  •  Q2 (c)  •  Q2 (d)  •  Q3 (a)  •  Q3 (b)  •  Q3 (c)  •  Q3 (d)  •  Q4 (a)  •  Q4 (b)  •  Q5 (a)  •  Q5 (b)  •  Q6  •  Q7 (a)  •  Q7 (b)  •  

   Other MATH101 Exams

  •  April 2011  •  April 2010  •  April 2009  •  April 2008  •  April 2007  •  April 2005  •  April 2006  •  April 2012  •  April 2013  •  April 2014  •  April 2015  •  April 2016  •  April 2018  •  April 2017  •  

Question 02 (c)
Full-Solution Problem. Justify your answers and show all your work. Simplification of answers is not required. Find the volume of the solid obtained by rotating the region bounded by the curves $y=5$ and $y=x+(4/x)$ about the line $x=-1$.

Make sure you understand the problem fully: What is the question asking you to do? Are there specific conditions or constraints that you should take note of? How will you know if your answer is correct from your work only? Can you rephrase the question in your own words in a way that makes sense to you?

If you are stuck, check the hint below. Consider it for a while. Does it give you a new idea on how to approach the problem? If so, try it!

Hint
Try using the method of cylindrical shells (shell integration).

Checking a solution serves two purposes: helping you if, after having used the hint, you still are stuck on the problem; or if you have solved the problem and would like to check your work. If you are stuck on a problem: Read the solution slowly and as soon as you feel you could finish the problem on your own, hide it and work on the problem. Come back later to the solution if you are stuck or if you want to check your work. If you want to check your work: Don't only focus on the answer, problems are mostly marked for the work you do, make sure you understand all the steps that were required to complete the problem and see if you made mistakes or forgot some aspects. Your goal is to check that your mental process was correct, not only the result.

Solution 1

Found a typo? Is this solution unclear? Let us know here. Please rate my easiness! It's quick and helps everyone guide their studies. The first step to solve this problem is to understand what volume we are trying to compute. The question states that there is a region bounded by the curves y=5 and y=x+4/x. For large values of x the second curve looks like the line y=x, but there is also a vertical asymptote at x=0. So it seems that it will indeed cross the horizontal line y=5 twice (as it dips down before going back up). We can compute where the intersections are by solving: $x+{\frac {4}{x}}=5$ which is equivalent to $\displaystyle 0=x^{2}-5x+4=(x-1)(x-4)$ and has solutions x=1 and x=4. Then we want to know how far down the curve goes, so we look for its local minimum. We compute its derivative: ${\frac {d}{dx}}\left(x+{\frac {4}{x}}\right)=1-{\frac {4}{x^{2}}}$ and hence see that it has two critical points, one at x=-2 and one at x=2. We are only interested in the local minimum which is at x=2. This should be enough details to have you be able to obtain the equivalent of the following picture: This in particular shows that y=5 is bigger than y=x+4/x. Now, consider a point x between 1 and 4. The distance from x to x=-1 is the radius of the cylindrical shell and is r=x+1. The height of the function at this point is the difference of the top function y=5 to the bottom function y=x+4/x which is 5-x-4/x. When we rotate, we travel a distance of $2\pi r$ (the circumference) and we are multiplying by $h\Delta x$. Thus, we have ${\begin{aligned}V&=\int _{1}^{4}2\pi (x+1)(5-x-4/x)\,dx\\&=2\pi \int _{1}^{4}(5x-x^{2}-4+5-x-4/x)\,dx\\&=2\pi \int _{1}^{4}(-x^{2}+4x+1-4/x)\,dx\\&=2\pi (-x^{3}/3+2x^{2}+x-4\ln |x|){\bigg |}_{1}^{4}\\&=2\pi ((-(4)^{3}/3+2(4)^{2}+4-4\ln |4|)-(-(1)^{3}/3+2(1)^{2}+1-4\ln |1|))\\&=2\pi ((-64/3+32+4-4\ln |4|)-(-1/3+2+1-0))\\&=2\pi (12-4\ln |4|)\\&=2\pi (12-8\ln(2))\\&=8\pi (3-2\ln(2))\\&=24\pi -16\pi \ln(2)\end{aligned}}$ Where each of the last four answers are equivalent and would be accepted as a final answer.

Solution 2

Found a typo? Is this solution unclear? Let us know here. Please rate my easiness! It's quick and helps everyone guide their studies. The first step to solve this problem is to understand what volume we are trying to compute. The question states that there is a region delimited by the curves y=5 and y=x+4/x. The second curve looks like the line y=x except that there is a vertical asymptote at x=0. So it seems that it will indeed cross the horizontal line y=5 twice (as it dips down before going back up). We can compute where the intersections are by solving: $x+{\frac {4}{x}}=5$ which is equivalent to $\displaystyle x^{2}-5x+4=0$ and has solutions x=1 and x=4. Then we want to know how far down the curve goes, so we look for its local minimum. We compute its derivative: ${\frac {d}{dx}}\left(x+{\frac {4}{x}}\right)=1-{\frac {4}{x^{2}}}$ and hence see that it has two critical points, one at x=-2 and one at x=2. We are only interested in the local minimum which is at x=2. 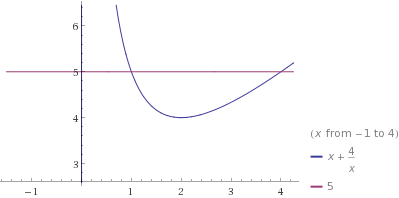This should be enough details to have you be able to obtain the equivalent of the following picture: Now we want to rotate this region around the vertical axis x=-1. To do this, we will use the method know as disk integration. The idea is fairly simple: imagine the solid viewed from the top (looking down at the axis), if we slice it along that axis, we will see a slice of a hollow cylinder. We will compute the area of that slice (the difference of area between the outer disk and the inner disk) and integrate. The outer disc has a radius that starts at the point (2,4) and moves right towards the point (4,5) following the curve y=x+4/x. The inner disc has a radius that starts at the point (2,4) and moves left towards the point (1,5) following the other side of the curve. We need to compute that radius (starting at x=-1). For this, the first step is to express the curve in terms of y instead of x: ${\begin{aligned}y=x+{\frac {4}{x}}&\iff yx=x^{2}+4\\&\iff x^{2}-yx+4=0\\&\iff x={\frac {y\pm {\sqrt {y^{2}-16}}}{2}}\end{aligned}}$ If we look at this, it makes sense: This is only well defined if y is larger than 4 (see on the graph) and there are two components: the one with the + for the right side and the one with the - for the left side. Since the axis of rotation is at x=-1, we need to add 1 to each of the radius and obtain: ${\begin{aligned}r_{outer}(y)&=1+{\frac {y+{\sqrt {y^{2}-16}}}{2}}\\r_{inner}(y)&=1+{\frac {y-{\sqrt {y^{2}-16}}}{2}}\end{aligned}}$ And so we can write the volume by integrating over all the shells, which are obtained by taking the difference of the outer and inner disk: $V=\int _{4}^{5}\left(\pi \left(1+{\frac {y}{2}}+{\frac {\sqrt {y^{2}-16}}{2}}\right)^{2}-\pi \left(1+{\frac {y}{2}}-{\frac {\sqrt {y^{2}-16}}{2}}\right)^{2}\right)\,dy$ (since the area of a disk of radius r is $\pi$ r2). Now we can do some algebra to rewrite this integral into something easier to handle. Notice the pattern here $\displaystyle (A+B)^{2}-(A-B)^{2}=4AB$ Hence we can rewrite this as ${\begin{aligned}V&=\pi \int _{4}^{5}4(1+{\frac {y}{2}})({\frac {\sqrt {y^{2}-16}}{2}})\,dy\\&=\pi \int _{4}^{5}(2+y)({\sqrt {y^{2}-16}})\,dy\\\end{aligned}}$ Now we need to compute that integral to obtain the desired volume. Unfortunately, this won't be straightforward since (2+y) isn't a multiple of the derivative of what is in the square root. There are many ways to compute this integral, here we will split it in 2 and deal with each term. $V=\pi \int _{4}^{5}2{\sqrt {y^{2}-16}}\,dy+\pi \int _{4}^{5}y{\sqrt {y^{2}-16}}\,dy$ Let us call the first integral V1 and the second one V2. The first one is the tougher one. Indeed: ${\begin{aligned}V_{2}&=\pi \int _{4}^{5}y{\sqrt {y^{2}-16}}\,dy\\&={\frac {\pi }{2}}\int _{4}^{5}2y{\sqrt {y^{2}-16}}\,dy\\&={\frac {\pi }{2}}\left[{\frac {2}{3}}(y^{2}-16)^{3/2}\right]_{4}^{5}\\&={\frac {\pi }{2}}{\frac {2}{3}}(25-16)^{3/2}\\&={\frac {\pi }{3}}\cdot 27\\&=9\pi \end{aligned}}$ The first integral is actually quite complicated. At the same time it is a classic. As of today (2012) you are not expected to be able to integrate this by hand and/or know the formula from memory. For completion sake, here is the formula: $\int {\sqrt {x^{2}-a^{2}}}\,dx={\frac {x}{2}}{\sqrt {x^{2}-a^{2}}}-{\frac {a^{2}}{2}}\ln(x+{\sqrt {x^{2}-a^{2}}})+c$ Which in this case gives us: ${\begin{aligned}V_{1}&=\pi \int _{4}^{5}2{\sqrt {y^{2}-16}}\,dy\\&=2\pi \left[{\frac {y}{2}}{\sqrt {y^{2}-16}}-8\ln(y+{\sqrt {y^{2}-16}})\right]_{4}^{5}\\&=2\pi \left[({\frac {5}{2}}{\sqrt {9}}-8\ln(5+{\sqrt {9}})-(0-8\ln(4+0))\right]\\&=2\pi ({\frac {15}{2}}-8\ln(8)+8\ln(4)\\&=\pi (15-48\ln(2)+32\ln(2))\\&=\pi (15-16\ln(2))\end{aligned}}$ Summing $\displaystyle V=V_{1}+V_{2}$ gives $\displaystyle V=24\pi -16\pi \ln(2)$ which is the same as above. ... This is why using the correct method of solids of revolution is very important.

Click here for similar questions

MER QGH flag, MER QGQ flag, MER QGS flag, MER QGT flag, MER Tag Solid of revolution, Pages using DynamicPageList3 parser function, Pages using DynamicPageList3 parser tag