|
As was suggested in the hint, one way to approach this problem is to draw a function that satisfies the first criterion and then modify it to fit each following criterion. It is worth noting that there are many, many ways to draw a picture satisfying all 4 criteria - the solution that follows is just one example.
Our first criterion is that the function be defined on the interval [-1,1]. We will draw just a straight line at  on this interval: on this interval:
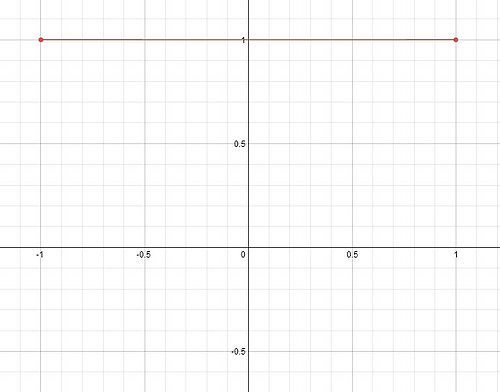
Now we add the criterion that the function be discontinuous at  and and  . One simple way to do this (without making the function undefined at those points, remember part 1!) is to move the two points at . One simple way to do this (without making the function undefined at those points, remember part 1!) is to move the two points at  and and  to a new location, in our case, the x-axis. to a new location, in our case, the x-axis.
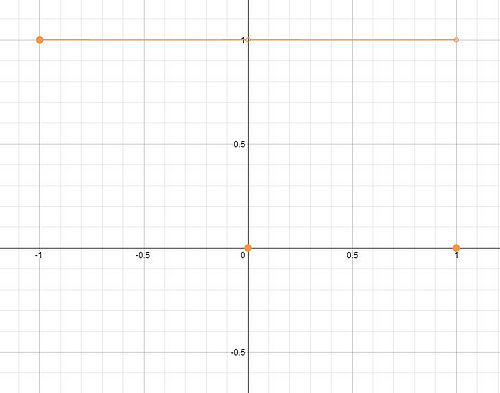
Now we want  to have a global minimum at to have a global minimum at  . This is easy enough - we'll just move our point that's now at the origin down until it is the lowest point on the entire graph. . This is easy enough - we'll just move our point that's now at the origin down until it is the lowest point on the entire graph.
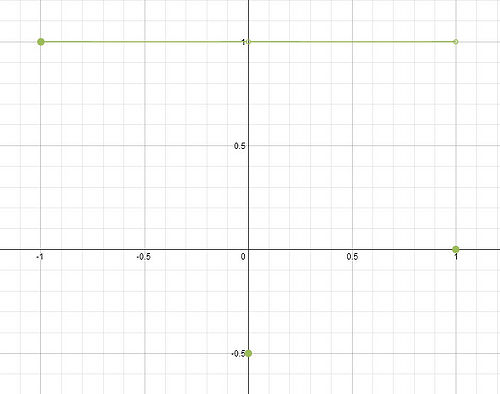
Finally, we can't have a local or global maximum. Right now, our straight line is both a local and global maximum because each point on the line is greater than or equal to its neighboring points. In order to make sure that each point on our line has a neighbor that is greater than itself, we will tilt the line, like so:
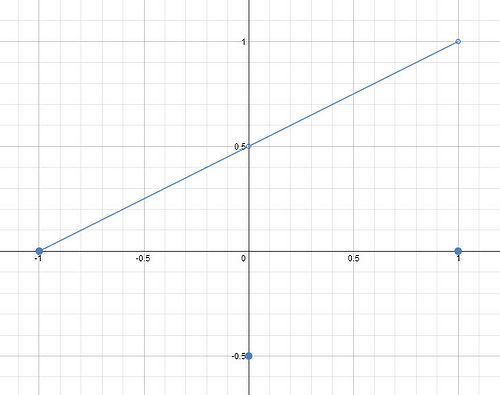
There are two important things to note here: first, this change has not altered any of the previous criteria (still defined on [-1,1], still discontinuous at appropriate points, etc.) Second, look at the right endpoint of the graph. Because this point is undefined,  cannot have a global or local maximum at this point, and any point on the line cannot be a maximum either. cannot have a global or local maximum at this point, and any point on the line cannot be a maximum either.
Thus we have drawn a function that satisfies all four criteria. We now simply translate our picture into a piecewise function. The "line" will be two pieces, and each point at  and and  will be another piece each. will be another piece each.

| 







