Science:Math Exam Resources/Courses/MATH101/April 2010/Question 04

MATH101 April 2010

• Q1 (a) • Q1 (b) • Q1 (c) • Q1 (d) • Q1 (e) • Q1 (f) • Q2 (a) • Q2 (b) • Q2 (c) • Q3 (a) • Q3 (b) • Q3 (c) • Q3 (d) • Q4 • Q5 (a) • Q5 (b) • Q5 (c) • Q6 • Q7 • Q8 • Q9 •

Other MATH101 Exams

• April 2011 • April 2010 • April 2009 • April 2008 • April 2007 • April 2005 • April 2006 • April 2012 • April 2013 • April 2014 • April 2015 • April 2016 • April 2018 • April 2017 •

[hide] Question 04
Full-Solution Problem. Justify your answer and show all your work. Simplification of the answer is not required. A colony of ants builds an anthill that is in the shape of a cone whose base, at ground level, is a circle of diameter 1 ft and whose height is also 1 ft. How much total work, in ft $\cdot$ lbs, is done by the ants in building the anthill? For the density of sand, use the value $150\,{\text{lb}}/{\text{ft}}^{3}.$

Make sure you understand the problem fully: What is the question asking you to do? Are there specific conditions or constraints that you should take note of? How will you know if your answer is correct from your work only? Can you rephrase the question in your own words in a way that makes sense to you?

If you are stuck, check the hint below. Consider it for a while. Does it give you a new idea on how to approach the problem? If so, try it!

[show] Hint
Split the cone up into cylinders with infinitely small height and add up the volume of these cylinders using integration.

Checking a solution serves two purposes: helping you if, after having used the hint, you still are stuck on the problem; or if you have solved the problem and would like to check your work.

If you are stuck on a problem: Read the solution slowly and as soon as you feel you could finish the problem on your own, hide it and work on the problem. Come back later to the solution if you are stuck or if you want to check your work.
If you want to check your work: Don't only focus on the answer, problems are mostly marked for the work you do, make sure you understand all the steps that were required to complete the problem and see if you made mistakes or forgot some aspects. Your goal is to check that your mental process was correct, not only the result.

[show] Solution
Consider the diagram for building the anthill . We can think of building the hill as stacking several small cylinders of height $\Delta {}x$ or ${\textrm {d}}x$ (thinking forward to integration) from the ground (x=1) to the top (x=0). Consider the anthill at some intermediate distance from the top x (at a distance 1-x from the ground). This point is represented in the diagram as $x_{i}^{}$ . 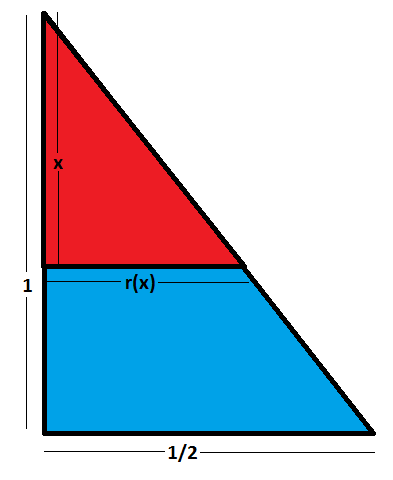The volume of a new cylinder to add onto the anthill will be $\displaystyle {}dV=\pi {}(r(x))^{2}{\textrm {d}}x$ where dx is the height of the cylinder and r(x)* is the radius of the anthill at the current height. There is a similar triangle problem between the top of the completed anthill and the current disk we're looking at Similar triangle problem for the anthill and so we can determine the radius at our current height x via ${\frac {1}{1/2}}={\frac {x}{r(x)}}$ and so $r(x)={\frac {x}{2}}.$ Notice that indeed when we're at the top (x=0) then the radius is zero and when we're at the bottom (x=1) that the radius is 1/2. We therefore have that the new amount of sand volume we are adding is $dV={\frac {\pi }{4}}x^{2}{\textrm {d}}x.$ In the problem we are given a density $150lb/ft^{3}$ which is actually a force density because lbs is a measure of force (note that this means, unlike for a mass density, we do not need to multiply by acceleration). This is telling us the force required to lift sand of a given volume which is precisely what we are trying to do with our cylinder of sand and so the force required to lift it is force = force density $\times$ volume $dF=150dV={\frac {150\pi }{4}}x^{2}{\textrm {d}}x.$ The work required to lift this cylinder to its position in the hill at x will be this force dF multiplied with the height we have to lift it from the ground 1-x. Therefore the amount of work to lift this cylinder is $dW=(1-x)dF={\frac {150\pi }{4}}x^{2}(1-x){\textrm {d}}x.$ This is the work to build one small cylinder. The whole hill is built by adding cylinders for all values of x and so to get the total work we integrate ${\begin{aligned}{\text{work}}=\int _{0}^{1}{\textrm {d}}W&=\int _{0}^{1}{\frac {150\pi }{4}}x^{2}\,(1-x)dx\\&=\left[{\frac {150\pi x^{3}}{12}}-{\frac {150\pi x^{4}}{16}}\right]_{0}^{1}\\&={\frac {150\pi }{12}}-{\frac {150\pi }{16}}\\&={\frac {25\pi }{8}}\end{aligned}}$ Let us do a quick sanity check on the units to see if we have missed out anything. The volume is in $ft^{3}$ , and the density is given in $lb/ft^{3}$ , so the weight is in $lb$ , finally work is force (weight) times distance (which is in $ft$ ), so we get our work to be in $ft\cdot lb$ , which is right. In conclusion, the work done by the ants to build the anthill is ${\frac {25\pi }{8}}ft\cdot lb$ .