Science:Math Exam Resources/Courses/MATH307/April 2013/Question Section 201 06 (a)

MATH307 April 2013

Work in progress: this question page is incomplete, there might be mistakes in the material you are seeing here.

  •  Q1 (a)  •  Q1 (b)  •  Q1 (c)  •  Q1 (d)  •  Q2 (a)  •  Q2 (b)  •  Q2 (c)  •  Q2 (d)  •  Q2 (e)  •  Q2 (f)  •  Q3 (a)  •  Q3 (b)  •  Q3 (c)  •  Q4 (a)  •  Q4 (b)  •  QS201 5(a)  •  QS201 5(b)  •  QS201 5(c)  •  QS201 6(a)  •  QS201 6(b)  •  QS201 6(c)  •  QS201 6(d)  •  QS201 7(a)  •  QS201 7(b)  •  QS201 7(c)  •  QS202 5(a)  •  QS202 5(b)  •  QS202 5(c)  •  QS202 5(d)  •  QS202 6(a)  •  QS202 6(b)  •  QS202 7(a)  •  QS202 7(b)  •  QS202 7(c)  •  QS202 7(d)  •  QS202 7(e)  •  

   Other MATH307 Exams

  •  December 2012  •  April 2013  •  April 2012  •  April 2006  •  December 2008  •  December 2010  •  

Question Section 201 06 (a)
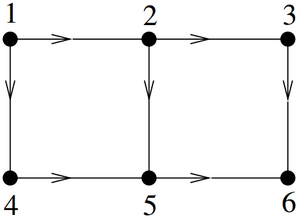
Consider the same graph as in the previous question, now interpreted as an internet where the vertices represent web pages and the arrows represent links. (a) Write down the stochastic matrix associated with the PageRank algorithm with no damping. Explain what you are doing with vertex 6.

Make sure you understand the problem fully: What is the question asking you to do? Are there specific conditions or constraints that you should take note of? How will you know if your answer is correct from your work only? Can you rephrase the question in your own words in a way that makes sense to you?

If you are stuck, check the hint below. Consider it for a while. Does it give you a new idea on how to approach the problem? If so, try it!

Hint
Suppose when on page i, that one can move to all pages i points to with equal probability. You are looking for the transition matrix, where component i of the state vector on which the matrix acts represents the probability of being at webpage i.

Checking a solution serves two purposes: helping you if, after having used the hint, you still are stuck on the problem; or if you have solved the problem and would like to check your work. If you are stuck on a problem: Read the solution slowly and as soon as you feel you could finish the problem on your own, hide it and work on the problem. Come back later to the solution if you are stuck or if you want to check your work. If you want to check your work: Don't only focus on the answer, problems are mostly marked for the work you do, make sure you understand all the steps that were required to complete the problem and see if you made mistakes or forgot some aspects. Your goal is to check that your mental process was correct, not only the result.

Solution

Found a typo? Is this solution unclear? Let us know here. Please rate my easiness! It's quick and helps everyone guide their studies. When $\alpha$ = 0, The stochastic matrix P is the sum of the outgoing links and the dangling links: $P={\begin{bmatrix}0&0&0&0&0&1/6\\1/2&0&0&0&0&1/6\\0&1/2&0&0&0&1/6\\1/2&0&0&0&0&1/6\\0&1/2&0&1&0&1/6\\0&0&1&0&1&1/6\end{bmatrix}}$ Vertex 6 has no links leaving the site, so it is a dangling node. When the web surfer reaches a dangling node, we assume that any page on the internet can be chosen at random with equal probability. This is why all the entries in column 6 of matrix P contain the value 1/6.