Science:Math Exam Resources/Courses/MATH307/April 2012/Question 04 (e)

MATH307 April 2012

Work in progress: this question page is incomplete, there might be mistakes in the material you are seeing here.

  •  Q1 (a)  •  Q1 (b)  •  Q1 (c)  •  Q1 (d)  •  Q2 (a)  •  Q2 (b)  •  Q2 (c)  •  Q3  •  Q4 (a)  •  Q4 (b)  •  Q4 (c)  •  Q4 (d)  •  Q4 (e)  •  Q5 (a)  •  Q5 (b)  •  Q5 (c)  •  Q6 (a)  •  Q6 (b)  •  Q6 (c)  •  Q6 (d)  •  Q7 (a)  •  Q7 (b)  •  Q7 (c)  •  Q8 (a)  •  Q8 (b)  •  Q8 (c)  •  

   Other MATH307 Exams

  •  December 2012  •  April 2013  •  April 2012  •  April 2006  •  December 2008  •  December 2010  •  

[hide] Question 04 (e)

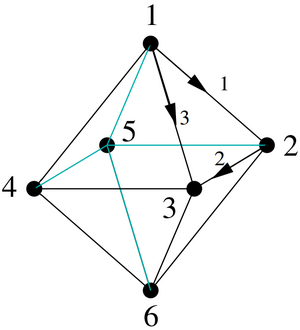
Consider a network arranged in the shape of an octahedron as in this diagram: The nodes have been labeled with the large numbers and 3 of the edges (resistors) have been given orientations and labeled with small numbers. Assume all resistances are equal to 1. Let D be the incidence matrix (for some choice of labeling and arrows for the remaining edges) and let L be the Laplacian. (e) It seems reasonable to conjecture that the effective resistance between nodes 1 and 6 would remain unchanged if removed the resistors between 2 and 3, 3 and 4, 4 and 5, 5 and 2. Explain how you could use MATLAB/Octave to test this conjecture. Assume that L has been defined in MATLAB/Octave.

Make sure you understand the problem fully: What is the question asking you to do? Are there specific conditions or constraints that you should take note of? How will you know if your answer is correct from your work only? Can you rephrase the question in your own words in a way that makes sense to you?

If you are stuck, check the hint below. Consider it for a while. Does it give you a new idea on how to approach the problem? If so, try it!

[show] Hint

Checking a solution serves two purposes: helping you if, after having used the hint, you still are stuck on the problem; or if you have solved the problem and would like to check your work. If you are stuck on a problem: Read the solution slowly and as soon as you feel you could finish the problem on your own, hide it and work on the problem. Come back later to the solution if you are stuck or if you want to check your work. If you want to check your work: Don't only focus on the answer, problems are mostly marked for the work you do, make sure you understand all the steps that were required to complete the problem and see if you made mistakes or forgot some aspects. Your goal is to check that your mental process was correct, not only the result.

[show] Solution

Found a typo? Is this solution unclear? Let us know here. Please rate my easiness! It's quick and helps everyone guide their studies. To find the effective resistance between two nodes, you would have to swap the 1st and sixth columns to the 1 and 2 positions inside the Laplacian matrix. You would then break up this Laplacian matrix into a block matrix: $L={\begin{bmatrix}A&B^{T}\\B&C\end{bmatrix}}$ where $A$ will be a 2x2 matrix. You would then find the voltage to current map (A.K.A Schur’s complement), matrix S whose equation is: $S=A-B^{T}C^{-1}B$ Then, after solving for S, the effective resistance would be the reciprocal of the 1st entry of matrix S. MATLAB CODE: %nodes n and m position n = 1; m = 6; %find the size of the matrix L lenL = length(L); %swap nodes n and m to the 1st and 2nd position in the Laplacian matrix swap = [n, m, 1:(n-1), (n+1):(m-1), (m+1):lenL]; L = L(swap, swap); %compute matrix S which is the voltage to current map (Schur's complement). A = L((1:2), (1:2)); B = L((3:lenL), (1:2)); C = L((3:lenL), (3:lenL)); S = A - (B’ * C(-1) * B); %finds the effective resistance which is the first entry of S. r = 1/S(1,1); You could run this for the 2 different Laplacian matrices to find the effective resistance for each and compare them to confirm the conjecture is correct.