|
The volume of the snowman is the volume of the body  , the volume of the head , the volume of the head  minus the volume of the intesection minus the volume of the intesection  . .
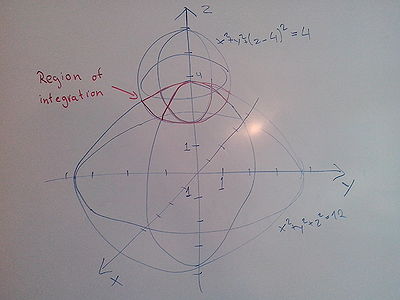
First we calculate  , which is the volume of the intersection of the two balls.
The intersection is bounded below from the surface , which is the volume of the intersection of the two balls.
The intersection is bounded below from the surface  and above from and above from  .
Since this shape is radially symmetric to the .
Since this shape is radially symmetric to the  -axes, we change to cylindrical coordinates.
This means -axes, we change to cylindrical coordinates.
This means  and and  . .
We find the intersection of the surfaces  and and  to find the upper bound for the radius to find the upper bound for the radius  : :

which yields  and and  . .
With these bounds we write  as as
 . .
Recall that the volume factor for cylindrical coordinates is  . .
![{\displaystyle \displaystyle {\begin{aligned}\iiint _{V_{i}}1\;{\text{d}}V&=\int _{0}^{\sqrt {3}}\int _{4-{\sqrt {4-r^{2}}}}^{\sqrt {12-r^{2}}}\int _{0}^{2\pi }r{\text{d}}\phi {\text{d}}z{\text{d}}r\\&=\int _{0}^{\sqrt {3}}2\pi r({\sqrt {12-r^{2}}}-4+{\sqrt {4-r^{2}}})\;{\text{d}}r\\&=\pi \left[-{\frac {2}{3}}(12-r^{2})^{\frac {3}{2}}-4r^{2}-{\frac {2}{3}}(4-r^{2})^{\frac {3}{2}}\right]_{0}^{\sqrt {3}}\\&=\pi \left[-{\frac {2}{3}}9^{\frac {3}{2}}-12-{\frac {2}{3}}+{\frac {2}{3}}12^{\frac {3}{2}}+0+{\frac {2}{3}}4^{\frac {3}{2}}\right]=\pi {\frac {4}{3}}(12{\sqrt {3}}-19)\end{aligned}}}](https://wiki.ubc.ca/api/rest_v1/media/math/render/svg/44fbd95beb4749109ec36d4b8e13727565a7cf84)
The ball  which makes the body has volume which makes the body has volume

The ball  which makes the head has volume which makes the head has volume

Hence, the volume of the snowman is

| 


















![{\displaystyle \displaystyle {\begin{aligned}\iiint _{V_{i}}1\;{\text{d}}V&=\int _{0}^{\sqrt {3}}\int _{4-{\sqrt {4-r^{2}}}}^{\sqrt {12-r^{2}}}\int _{0}^{2\pi }r{\text{d}}\phi {\text{d}}z{\text{d}}r\\&=\int _{0}^{\sqrt {3}}2\pi r({\sqrt {12-r^{2}}}-4+{\sqrt {4-r^{2}}})\;{\text{d}}r\\&=\pi \left[-{\frac {2}{3}}(12-r^{2})^{\frac {3}{2}}-4r^{2}-{\frac {2}{3}}(4-r^{2})^{\frac {3}{2}}\right]_{0}^{\sqrt {3}}\\&=\pi \left[-{\frac {2}{3}}9^{\frac {3}{2}}-12-{\frac {2}{3}}+{\frac {2}{3}}12^{\frac {3}{2}}+0+{\frac {2}{3}}4^{\frac {3}{2}}\right]=\pi {\frac {4}{3}}(12{\sqrt {3}}-19)\end{aligned}}}](https://wiki.ubc.ca/api/rest_v1/media/math/render/svg/44fbd95beb4749109ec36d4b8e13727565a7cf84)


