Science:Math Exam Resources/Courses/MATH103/April 2011/Question 04 (b)

{{#incat:MER QGQ flag|{{#incat:MER QGH flag|{{#incat:MER QGS flag|}}}}}}

MATH103 April 2011

  •  Q1 (a)  •  Q1 (b)  •  Q1 (c)  •  Q2 (a)  •  Q2 (b)  •  Q2 (c)  •  Q2 (d)  •  Q3 (a)  •  Q3 (b)  •  Q4 (a)  •  Q4 (b)  •  Q5 (a)  •  Q5 (b)  •  Q5 (c)  •  Q5 (d)  •  Q6 (a)  •  Q6 (b)  •  Q7 (a)  •  Q7 (b)  •  Q7 (c)  •  Q8  •  

   Other MATH103 Exams

  •  April 2006  •  April 2005  •  April 2011  •  April 2010  •  April 2009  •  April 2012  •  April 2013  •  April 2014  •  April 2015  •  April 2016  •  April 2017  •  

Question 04 (b)
Consider the function $f(x)=e^{x}$ for 0 ≤ x ≤ 1. Find the volume $V_{2}$ of the bowl when rotating $f(x)$ around the $y$-axis. (Note: for this problem there are two ways to set the relevant integral up - either one is fine. One way involves rewriting $f(x)$ as $x=g(y)$.)

Make sure you understand the problem fully: What is the question asking you to do? Are there specific conditions or constraints that you should take note of? How will you know if your answer is correct from your work only? Can you rephrase the question in your own words in a way that makes sense to you?

If you are stuck, check the hints below. Read the first one and consider it for a while. Does it give you a new idea on how to approach the problem? If so, try it! If after a while you are still stuck, go for the next hint.

Hint 1
Draw a picture. Visualize the two ways of integrating as referred to in the hint.

Hint 2
Write down two integrals that represent the volume $V_{2}$ of the solid. Which one would you prefer to evaluate?

Hint 3
In this case, both integrals are possible to evaluate using the techniques that you've learned. It is a good reality check (and also a good exercise) to compute both integrals and ensure that you obtain the same result.

Checking a solution serves two purposes: helping you if, after having used all the hints, you still are stuck on the problem; or if you have solved the problem and would like to check your work. If you are stuck on a problem: Read the solution slowly and as soon as you feel you could finish the problem on your own, hide it and work on the problem. Come back later to the solution if you are stuck or if you want to check your work. If you want to check your work: Don't only focus on the answer, problems are mostly marked for the work you do, make sure you understand all the steps that were required to complete the problem and see if you made mistakes or forgot some aspects. Your goal is to check that your mental process was correct, not only the result.

Solution 1

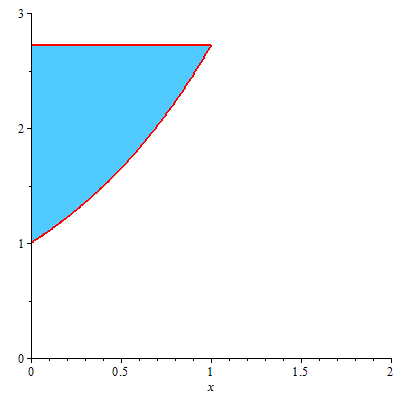
Disc Method. In this solution, we consider the volume as a sum of slices, cut orthogonal to the $y$-axis. Each slice has volume $\pi r^{2}\Delta y$, where $r$ is its radius and $\Delta y$ is its thickness. The bowl will be made by rotating the shaded blue region in the 2D figure xy 2D image . If the slice is at height $y$, then its radius is equal to $x$, where $y=e^{x}$. Hence, $r=\ln y.$ In the limit $\Delta y\to 0,$ ${\begin{aligned}V_{2}=\int _{1}^{e}\pi (\ln y)^{2}\,dy\end{aligned}}$ Note that the limits of integration are $f(0)=e^{0}=1$ and $f(1)=e^{1}=e,$ since $x$ ranges from 0 to 1. We use integration by parts to evaluate this integral, letting $f(y)=g(y)=\ln y.$ So in order to perform the integration by parts we need to recall the antiderivative of $\ln y$. To calculate this antiderivative we again use integration by parts: ${\begin{aligned}\int \ln ydy&=\int 1\cdot \ln y\,dy=y\ln y-\int y\cdot {\frac {1}{y}}\,dy\\&=y\ln y-\int 1\,dy=y\ln y-y+C.\end{aligned}}$ We now use this antiderivative to perform the integration by parts in the integral for $V_{2}.$ Hence, ${\begin{aligned}V_{2}&=\pi \int _{1}^{e}(\ln y)^{2}\,dy\\&=\pi \left[\ln y(y\ln y-y)\right]_{1}^{e}-\pi \int _{1}^{e}{\frac {1}{y}}(y\ln y-y)\,dy\\&=\pi \left[1(e\cdot 1-e)-0\right]-\pi \int _{1}^{e}(\ln y-1)\,dy\\&=-\pi \left[y\ln y-y-y\right]_{1}^{e}\\&=-\pi \left[e\ln e-e-e-1\ln 1+1+1\right]\\&=-\pi \left(-e+2\right)\\&=\pi \left(e-2\right).\\\end{aligned}}$ Reality check: Note that we obtain the same value for $V_{2}$ with both methods. To visualize the full bowl we can look at the 3D figure 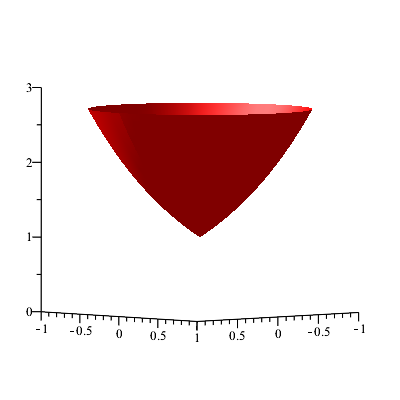Full 3D image from rotation .

Solution 2

Shell method. In this solution, we consider the solid to be a sum of cylindrical shells. Each cylindrical shell has volume $2\pi r(x)h(x)\Delta r,$ where $r(x)$ is its radius, $h(x)$ is its height and $\Delta r$ is its thickness. The bowl will be made up by rotating the blue shaded region in the 2D figure xy 2D image . From there we have $r(x)=x$, $h(x)=f(1)-f(x)=e-e^{x},$ and $\Delta r=\Delta x$. In the limit $\Delta x\to 0,$ we obtain the volume by integrating along the x-axis: ${\begin{aligned}V_{2}&=\int _{0}^{1}2\pi r(x)h(x)\,dx\\&=\int _{0}^{1}2\pi x(e-e^{x})\,dx\\&=2\pi e\int _{0}^{1}x\,dx-2\pi \int _{0}^{1}xe^{x}\,dx\\&=2\pi e\left[{\frac {x^{2}}{2}}\right]_{0}^{1}-2\pi \left(\left[xe^{x}\right]_{0}^{1}-\int _{0}^{1}e^{x}\,dx\right)\\&=2\pi e{\frac {1}{2}}-2\pi \left(e-\left[e^{x}\right]_{0}^{1}\right)\\&=\pi e-2\pi \left(e-(e-1)\right)\\&=\pi e-2\pi \\&=\pi (e-2).\end{aligned}}$ (We used integration by parts in the second integral to get from the third to the fourth line.) Reality check: Note that we obtain the same value for $V_{2}$ with both methods. To visualize the full bowl we can look at the 3D figure Full 3D image from rotation .

{{#incat:MER CT flag||

Click here for similar questions

MER QGH flag, MER QGQ flag, MER QGS flag, MER RT flag, MER Tag Solid of revolution, Pages using DynamicPageList3 parser function, Pages using DynamicPageList3 parser tag

}}