Science:Math Exam Resources/Courses/MATH103/April 2011/Question 03 (a)

MATH103 April 2011

  •  Q1 (a)  •  Q1 (b)  •  Q1 (c)  •  Q2 (a)  •  Q2 (b)  •  Q2 (c)  •  Q2 (d)  •  Q3 (a)  •  Q3 (b)  •  Q4 (a)  •  Q4 (b)  •  Q5 (a)  •  Q5 (b)  •  Q5 (c)  •  Q5 (d)  •  Q6 (a)  •  Q6 (b)  •  Q7 (a)  •  Q7 (b)  •  Q7 (c)  •  Q8  •  

   Other MATH103 Exams

  •  April 2006  •  April 2005  •  April 2011  •  April 2010  •  April 2009  •  April 2012  •  April 2013  •  April 2014  •  April 2015  •  April 2016  •  April 2017  •  

Question 03 (a)
Consider the function $f(x)=x(x-2)(x+1)$. Sketch the function.

Make sure you understand the problem fully: What is the question asking you to do? Are there specific conditions or constraints that you should take note of? How will you know if your answer is correct from your work only? Can you rephrase the question in your own words in a way that makes sense to you?

If you are stuck, check the hint below. Consider it for a while. Does it give you a new idea on how to approach the problem? If so, try it!

Hint
What are the roots of $f$? What happens to $f(x)$ when $x\rightarrow \pm \infty$? In which intervals is $f(x)$ positive, in which is the function negative? Find any local maxima and local minima.

Checking a solution serves two purposes: helping you if, after having used the hint, you still are stuck on the problem; or if you have solved the problem and would like to check your work. If you are stuck on a problem: Read the solution slowly and as soon as you feel you could finish the problem on your own, hide it and work on the problem. Come back later to the solution if you are stuck or if you want to check your work. If you want to check your work: Don't only focus on the answer, problems are mostly marked for the work you do, make sure you understand all the steps that were required to complete the problem and see if you made mistakes or forgot some aspects. Your goal is to check that your mental process was correct, not only the result.

Solution

Found a typo? Is this solution unclear? Let us know here. Please rate my easiness! It's quick and helps everyone guide their studies. We will preface this question by noting that the original intent in the exam was to understand the sketch well enough to proceed to part (b). However, we have decided to present the solution as detailed as possible for those who would like to improve their curve sketching techniques. Now we will complete this question by following a checklist that is fairly standard for these types of problems. Find roots Determine any asymptotes Determine critical points Determine intervals of increase and decrease and local max/min Determine intervals of concavity and points of inflection Sketch the graph 1: Find roots: Luckily we have a polynomial that is already written in factored form so we can read, as the roots, the factors. Therefore if $\displaystyle {}f(x)=x(x-2)(x+1)=0$ then the roots are at $x=0$, $x=2$, $x=-1$. 2: Asymptotes: Now polynomials are continuous everywhere (their domain is all the real numbers) and so we do not expect any vertical asymptotes. When looking for horizontal asymptotes we typically take the limit as $x$ goes to $\infty$. We don't need to be so formal here because it's clear that as $x$ gets large then $f(x)$ also gets large as their is nothing (such as a denominator) to slow its growth. What's to be determined is whether $f(x)$ gets large and positive or large and negative. If we were to put a very large positive value of $x$ into $f(x)$ we would get that $f(x)=x(x-2)(x+1)\approx {}x^{3}$ since adding or subtracting numbers to something large is unaffected. Now as $x$ goes to $\infty$ then $f(x)$ will go to $\infty$ as well. If we put in a large negative number, once again adding or subtracting small numbers will have no affect and once again, $f(x)\approx {}x^{3}$ which approaches $-\infty$ as $x$ goes to $-\infty$. Therefore, ${\begin{aligned}\lim _{x\to \infty }f(x)&=+\infty \\\lim _{x\to {-\infty }}f(x)&=-\infty .\end{aligned}}$ 3. Critical Points: In order to find critical points we first need the derivative. In order to do this we need to apply the product rule three times. Therefore, $\displaystyle {}f'(x)=(x-2)(x+1)+x(x+1)+x(x-2)=3x^{2}-2x-2.$ Critical points will occur when $f'(x)=0$. Therefore, ${\begin{aligned}3x^{2}-2x-2&=0\\x={\frac {2\pm {\sqrt {4+24}}}{6}}&={\frac {1}{3}}\pm {\frac {\sqrt {7}}{3}}\end{aligned}}$ are our critical points. 4. Intervals of Increase and Decrease and Local Max/Min: We have that our derivative is, $\displaystyle {}f'(x)=3x^{2}-2x-2$ with critical points $x={\frac {1}{3}}\pm {\frac {\sqrt {7}}{3}}.$ To find intervals of increasing and decreasing we look at the derivative between each critical point over the entire domain. If the derivative is positive then the function is increasing and if the derivative is negative, the function is decreasing. $\left(-\infty ,{\frac {1}{3}}-{\frac {\sqrt {7}}{3}}\right)$ $\left({\frac {1}{3}}-{\frac {\sqrt {7}}{3}},{\frac {1}{3}}+{\frac {\sqrt {7}}{3}}\right)$ $\left({\frac {1}{3}}+{\frac {\sqrt {7}}{3}},\infty \right)$ $\displaystyle {}f'(x)$ Positive Negative Positive $\displaystyle {}f(x)$ Increasing Decreasing Increasing From the table we see that the critical point ${\frac {1}{3}}-{\frac {\sqrt {7}}{3}}$ is a local maximum since the derivative is increasing on the left and decreasing on the right. By a similar logic we have that the other critical point ${\frac {1}{3}}+{\frac {\sqrt {7}}{3}}$ is a local minimum. 5. Intervals of Concavity and Points of Inflection: In order to determine points of inflection we have to compute the second derivative. Since the first derivative is just a polynomial we can easily compute term by term with the power rule to get $\displaystyle {}f''(x)=6x-2.$ Possible points of inflection occur when $f''(x)=0$, i.e. there is a possible point of inflection as $x=1/3$. In order for it to be a point of inflection we require that the second derivative change sign as we cross $x=1/3$. If the second derivative is positive, the graph is concave up; if it is negative, the graph is concave down. $\left(-\infty ,{\frac {1}{3}}\right)$ $\left({\frac {1}{3}},\infty \right)$ $\displaystyle {}f''(x)$ Negative Positive $\displaystyle {}f(x)$ Concave Down Concave Up Notice that the change in concavity, confirms that we do indeed have an inflection point at $x=1/3$. 6. 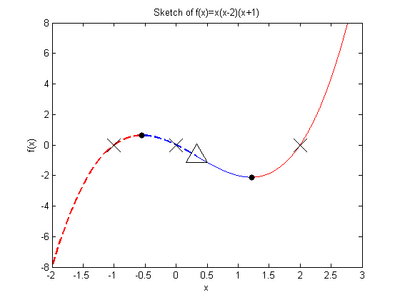Sketch: The picture to the right shows a sketch of the graph. We have labelled intervals of increasing with red and decreasing with blue. Concave up is a thin solid line while concave down is a thick dashed line. We have placed x's to mark the roots, filled in circles to mark the local maximum and minimum, and triangles to indicate the point of inflection.

Click here for similar questions

MER QGH flag, MER QGQ flag, MER QGS flag, MER RT flag, MER Tag Graphing of a function, Pages using DynamicPageList3 parser function, Pages using DynamicPageList3 parser tag