Science:Math Exam Resources/Courses/MATH100 A/December 2023/Question 12

MATH100 A December 2023

Work in progress: this question page is incomplete, there might be mistakes in the material you are seeing here.

  •  Q1  •  Q2  •  Q3  •  Q4  •  Q5  •  Q6  •  Q7  •  Q8  •  Q9  •  Q10  •  Q11  •  Q12  •  Q13  •  Q14  •  Q15  •  Q16  •  Q17  •  Q18  •  Q19  •  Q20  •  Q21  •  Q22  •  Q23  •  Q24  •  Q25  •  Q26  •  Q27(a)  •  Q27(b)  •  Q27(c)  •  Q28(a)  •  Q28(b)  •  Q29(a)  •  Q29(b)  •  Q30  •  

   Other MATH100 A Exams

  •  December 2023  •  

[hide] Question 12
At noon, ship ${\textstyle A}$ is 4km west of ship ${\textstyle B}$. Ship ${\textstyle A}$ is sailing south at 2km/h and ship ${\textstyle B}$ is sailing north at 4km/h. The scenario some time after noon is pictured below. How fast is the distance between the ships changing 30 minutes after noon? Diagram of boats.

Make sure you understand the problem fully: What is the question asking you to do? Are there specific conditions or constraints that you should take note of? How will you know if your answer is correct from your work only? Can you rephrase the question in your own words in a way that makes sense to you?

If you are stuck, check the hint below. Consider it for a while. Does it give you a new idea on how to approach the problem? If so, try it!

[show] Hint

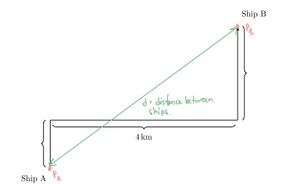
We need to come up with an expression that represents the distance between Ship A and Ship B. Let ${\textstyle d}$ be the distance between Ship A and Ship B, and say that “up” is the positive direction, and at noon when the ships are 4km apart, the ${\textstyle y}$-coordinate of their position is 0. Looking at the upper figure, we could re-arrange the paths of ships A and B to create a right-angle triangle (lower figure). 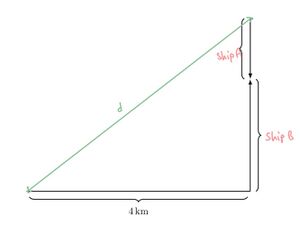distance between ships distance between ships rearranged

Checking a solution serves two purposes: helping you if, after having used the hint, you still are stuck on the problem; or if you have solved the problem and would like to check your work. If you are stuck on a problem: Read the solution slowly and as soon as you feel you could finish the problem on your own, hide it and work on the problem. Come back later to the solution if you are stuck or if you want to check your work. If you want to check your work: Don't only focus on the answer, problems are mostly marked for the work you do, make sure you understand all the steps that were required to complete the problem and see if you made mistakes or forgot some aspects. Your goal is to check that your mental process was correct, not only the result.

[show] Solution

We need to come up with an expression that represents the distance between Ship A and Ship B. Let ${\textstyle d}$ be the distance between Ship A and Ship B, and say that “up” is the positive direction, and at noon when the ships are 4km apart, the ${\textstyle y}$-coordinate of their position is 0. Looking at the upper figure, we could re-arrange the paths of ships A and B to create a right-angle triangle (lower figure). distance between ships distance between ships rearranged Using this, we can write an expression for ${\textstyle d}$ that depends on the ${\textstyle y}$-coordinate of the positions of ships A and B: $$d={\sqrt {4^{2}+(p_{B}-p_{A})^{2}}}$$ To determine how fast the distance between the ships is changing, we take the derivative of ${\textstyle d}$ with respect to time. $${\begin{aligned}{\frac {d}{dt}}d&={\frac {d}{dt}}{\sqrt {4^{2}+(p_{B}-p_{A})^{2}}}\\&={\frac {1}{2}}(16+(p_{B}-p_{A})^{2})^{-1/2}\left(2(p_{B}-p_{A})({\frac {dp_{B}}{dt}}-{\frac {dp_{A}}{dt}})\right)\\&={\frac {(p_{B}-p_{A})({\frac {dp_{B}}{dt}}-{\frac {dp_{A}}{dt}})}{\sqrt {16+(p_{B}-p_{A})^{2}}}}\end{aligned}}$$ So, now we need to determine ${\textstyle p_{A}}$, ${\textstyle p_{B}}$, ${\textstyle {\frac {dp_{A}}{dt}}}$, and ${\textstyle {\frac {dp_{B}}{dt}}}$ 30mins after noon. Say at noon, time ${\textstyle t=0}$ and measure time in hours. Then, 30mins later ${\textstyle t=0.5}$. We know ship A and ship B are travelling at a constant speed, so ${\textstyle {\frac {dp_{A}}{dt}}=-2}$ km/h and ${\textstyle {\frac {dp_{B}}{dt}}=4}$ km/h. The ${\textstyle y}$-coordinate of their positions can be determined using ${\textstyle speed={\frac {distance}{time}}}$, so: $${\begin{aligned}p_{A}(0.5)&=(-2km/h)(0.5h)=-1km\\p_{B}(0.5)&=(4km/h)(0.5h)=2km\end{aligned}}$$ Now we can calculate how fast the distance between the ships is changing: $${\begin{aligned}d(0.5h)&={\frac {(2-(-1))(4-(-2))}{\sqrt {16+(2-(-1))^{2}}}}\\&={\frac {18}{5}}\end{aligned}}$$ Therefore, the distance between the ships is changing at ${\textstyle {\frac {18}{5}}}$ km/h.