|
In short, the Fourier convergence theorem states the following.
If  is a periodic function such that is a periodic function such that  and and  are piecewise continuous, then the Fouriner series converges to are piecewise continuous, then the Fouriner series converges to  at all points where at all points where  is continuous and converges to is continuous and converges to ![{\displaystyle {\frac {1}{2}}[f(x+)+f(x-)]}](https://wiki.ubc.ca/api/rest_v1/media/math/render/svg/ed90fb492f4e27ef2ea6c4ec9ebe8a1ecdd9a52c) at all points where at all points where  is discontinuous. is discontinuous. |
In the above,  denotes the right hand limit of denotes the right hand limit of  at at  , while , while  denotes the left hand limit of denotes the left hand limit of  at at  . In other words, for a real number . In other words, for a real number  , ,

With the Fourier convergence theorem in mind, let's find out the point of convergence of the Fourier cosine series  at each point at each point  in the interval in the interval ![{\displaystyle [0,1]}](https://wiki.ubc.ca/api/rest_v1/media/math/render/svg/738f7d23bb2d9642bab520020873cccbef49768d) . To do so, we first need to understand the origin of the Fourier cosine series. Recall that the Fourier cosine series is the Fourier series of . To do so, we first need to understand the origin of the Fourier cosine series. Recall that the Fourier cosine series is the Fourier series of  where where  is the even extension of is the even extension of  . Following the Fourier convergence theorem, to find the point of convergence of the Fourier cosine series, we need to know where the points of continuity and discontinuity of . Following the Fourier convergence theorem, to find the point of convergence of the Fourier cosine series, we need to know where the points of continuity and discontinuity of  are. We will do so by sketching the graph of are. We will do so by sketching the graph of  . To begin, we sketch the graph of . To begin, we sketch the graph of  . .
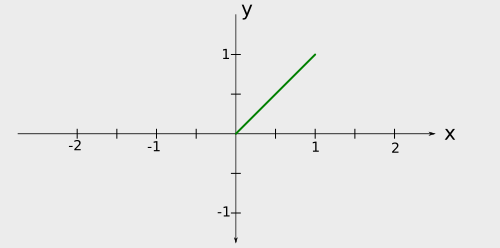 A sketch of  The graph of  is obtained by first reflecting is obtained by first reflecting  over the y-axis and hence obtaining a function over the interval over the y-axis and hence obtaining a function over the interval ![{\displaystyle [-1,1]}](https://wiki.ubc.ca/api/rest_v1/media/math/render/svg/51e3b7f14a6f70e614728c583409a0b9a8b9de01) .
We then periodic extend this function to get .
We then periodic extend this function to get  which will have a period of which will have a period of  . .
 A sketch of the even extension of  . The part of the function from  to  is coloured in red. The red part shows one period of  .
From the sketch of  , we see that , we see that  is continuous everywhere. Hence, by the Fourier convergence theorem, the Fourier cosine series is continuous everywhere. Hence, by the Fourier convergence theorem, the Fourier cosine series  converges to converges to  for every real number for every real number  . Now, since . Now, since  agrees with agrees with  at every point in the interval at every point in the interval ![{\displaystyle [0,1]}](https://wiki.ubc.ca/api/rest_v1/media/math/render/svg/738f7d23bb2d9642bab520020873cccbef49768d) , ,
 converges to converges to  at every point at every point  in the interval in the interval ![{\displaystyle [0,1]}](https://wiki.ubc.ca/api/rest_v1/media/math/render/svg/738f7d23bb2d9642bab520020873cccbef49768d) . .
Similarly, let  be the odd extension of be the odd extension of  . Then the Fourier sine series . Then the Fourier sine series  is the Fourier series of is the Fourier series of  . We will sketch . We will sketch  to find its points of continuity and discontinuity. to find its points of continuity and discontinuity.
The graph of  is obtained by first rotating is obtained by first rotating  about the origin by 180 degree and hence obtaining a function over the interval about the origin by 180 degree and hence obtaining a function over the interval ![{\displaystyle [-1,1]}](https://wiki.ubc.ca/api/rest_v1/media/math/render/svg/51e3b7f14a6f70e614728c583409a0b9a8b9de01) . We then periodic extend this function to get . We then periodic extend this function to get  which will have a period of which will have a period of  . .
 A sketch of the odd extension of  . The part of the function from  to  is coloured in red. The red part shows one period of  .The values at points of discontinuity is not specified in the sketch. From the sketch of  is discontinuous at points is discontinuous at points  with k an integer. Hence, by the Fourier convergence theorem, the Fourier sine series with k an integer. Hence, by the Fourier convergence theorem, the Fourier sine series  converges to converges to  at every point in the interval at every point in the interval  , but at , but at  , ,  converges to converges to
![{\displaystyle {\frac {1}{2}}[f_{\text{odd}}(1-)+f_{\text{odd}}(1+)]={\frac {1}{2}}[1+(-1)]=0}](https://wiki.ubc.ca/api/rest_v1/media/math/render/svg/e5be62c931e1c3a72d0dea65bd3c3597ff12c637) . .
Since  agrees with agrees with  in the interval in the interval  , ,
-
 converges to converges to  at every point in the interval at every point in the interval  and at and at  , ,  converges to 0. converges to 0.
Finally, the question asks us to verify our conclusions for the Fourier sine series at x = 0 and x = 1. Evaluate the sine series found at part a at  , we get , we get

and evaluating at  , we get , we get

as expected.
|






![{\displaystyle {\frac {1}{2}}[f(x+)+f(x-)]}](https://wiki.ubc.ca/api/rest_v1/media/math/render/svg/ed90fb492f4e27ef2ea6c4ec9ebe8a1ecdd9a52c)





![{\displaystyle [0,1]}](https://wiki.ubc.ca/api/rest_v1/media/math/render/svg/738f7d23bb2d9642bab520020873cccbef49768d)


![{\displaystyle [-1,1]}](https://wiki.ubc.ca/api/rest_v1/media/math/render/svg/51e3b7f14a6f70e614728c583409a0b9a8b9de01)











![{\displaystyle {\frac {1}{2}}[f_{\text{odd}}(1-)+f_{\text{odd}}(1+)]={\frac {1}{2}}[1+(-1)]=0}](https://wiki.ubc.ca/api/rest_v1/media/math/render/svg/e5be62c931e1c3a72d0dea65bd3c3597ff12c637)



