Science:Math Exam Resources/Courses/MATH105/April 2011/Question 09 (e)

MATH105 April 2011

  •  Q1 (a)  •  Q1 (b)  •  Q2  •  Q3  •  Q4  •  Q5 (a)  •  Q5 (b)  •  Q5 (c)  •  Q6  •  Q7  •  Q8 (a)  •  Q8 (b)  •  Q8 (c)  •  Q9 (a)  •  Q9 (b)  •  Q9 (c)  •  Q9 (d)  •  Q9 (e)  •  Q9 (f)  •  Q9 (g)  •  

   Other MATH105 Exams

  •  April 2009  •  April 2011  •  April 2010  •  April 2012  •  April 2013  •  April 2014  •  April 2015  •  April 2016  •  April 2017  •  April 2018  •  

Question 09 (e)

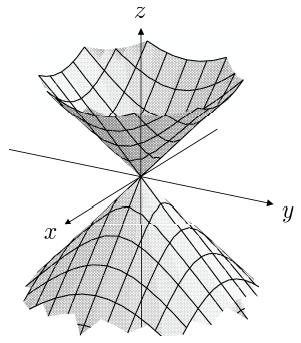
Each of the short-answer questions below is worth 5 points. Put your answer in the box provided and show your work. No credit will be given for the answer without the correct accompanying work. In the box, write down which of the equations $z=x^{2}+y^{2},\quad z=x^{2}-y^{2},\quad x^{2}+y^{2}+z^{2}=1,\quad z^{2}=x^{2}+y^{2}$ describes the surface with the following diagram? If $z=f(x,y)$ is the equation of the surface in the diagram, sketch in the space provided below the level curve of $f$ at height $z=2.$ Provided explicit labels for your sketch.

Make sure you understand the problem fully: What is the question asking you to do? Are there specific conditions or constraints that you should take note of? How will you know if your answer is correct from your work only? Can you rephrase the question in your own words in a way that makes sense to you?

If you are stuck, check the hint below. Consider it for a while. Does it give you a new idea on how to approach the problem? If so, try it!

Hint
Try to rule out some possibilities by considering whether the 4 functions given satisfy some basic properties which the surface clearly satisfies, i.e.: the point (0,0,0) is on the surface, the surface takes on negative $z$ values and is symmetric about the $x,y$ plane.

Checking a solution serves two purposes: helping you if, after having used the hint, you still are stuck on the problem; or if you have solved the problem and would like to check your work. If you are stuck on a problem: Read the solution slowly and as soon as you feel you could finish the problem on your own, hide it and work on the problem. Come back later to the solution if you are stuck or if you want to check your work. If you want to check your work: Don't only focus on the answer, problems are mostly marked for the work you do, make sure you understand all the steps that were required to complete the problem and see if you made mistakes or forgot some aspects. Your goal is to check that your mental process was correct, not only the result.

Solution

Found a typo? Is this solution unclear? Let us know here. Please rate my easiness! It's quick and helps everyone guide their studies. As the hint suggests, we can check each equation with the point (0,0,0), which lies on the surface. The point (0,0,0) does not lie on the surface given by $x^{2}+y^{2}+z^{2}=1$, so we can rule that out. Since the surface takes negative $z$ values and $z=x^{2}+y^{2}$ only takes non-negative values, we can rule this out as an option as well. Finally, we notice that the surface is symmetric about the $x,y$ plane (this means that for a given (x,y) we get a value z above the xy-plane and the same value (but negative), -z below the xy-plane. For this reason, we can rule out $z=x^{2}-y^{2}$ since for a given (x,y) we only produce one z value. Thus the surface is $z^{2}=x^{2}+y^{2}$. We can check that this solution makes sense by solving the equation for $z$ to get $z=\pm {\sqrt {x^{2}+y^{2}}}$. The two solutions give identical surfaces above and below the $x,y$ plane, the point (0,0,0) does indeed lie on the surface $z^{2}=x^{2}+y^{2}$, and as $x$ and $y$ increase, so does $z$. These all match the given graph. The level curve at $z=2$ is what we would see if we sliced into the surface horizontally at $z=2$ and looked at it from above. We can find the equation by substituting into the equation for the surface: $2^{2}=x^{2}+y^{2}$. This is simply a circle of radius $2$, the graph as follows:

Click here for similar questions

MER QGH flag, MER QGQ flag, MER QGS flag, MER RT flag, MER Tag Level curves, Pages using DynamicPageList3 parser function, Pages using DynamicPageList3 parser tag