Science:Math Exam Resources/Courses/MATH101/April 2014/Question 04

{{#incat:MER QGQ flag|{{#incat:MER QGH flag|{{#incat:MER QGS flag|}}}}}}

MATH101 April 2014

• Q1 (a) • Q1 (b) • Q1 (c) • Q1 (d) • Q1 (e) • Q1 (f) • Q1 (g) • Q1 (h) • Q1 (i) • Q1 (j) • Q1 (k) • Q2 • Q3 • Q4 • Q5 • Q6 • Q7 (a) • Q7 (b) • Q8 • Q9 (a) • Q9 (b) •

Other MATH101 Exams

• April 2011 • April 2010 • April 2009 • April 2008 • April 2007 • April 2005 • April 2006 • April 2012 • April 2013 • April 2014 • April 2015 • April 2016 • April 2018 • April 2017 •

Question 04
Long Problem. Show your work. No credit will be given for the answer without the correct accompanying work. A spherical tank of radius 3 metres is half-full of water. It has a spout of length 1 metre sticking up from the top of the tank. Find the work required to pump all of the water in the tank out the spout. The density of water is 1000 kilograms per cubic metre. The acceleration due to gravity is 9.8 metres per second squared. Spherical tank

Make sure you understand the problem fully: What is the question asking you to do? Are there specific conditions or constraints that you should take note of? How will you know if your answer is correct from your work only? Can you rephrase the question in your own words in a way that makes sense to you?

If you are stuck, check the hints below. Read the first one and consider it for a while. Does it give you a new idea on how to approach the problem? If so, try it! If after a while you are still stuck, go for the next hint.

Hint 1
Work is defined as acceleration ( $a$ ) multiplied by displacement ( $h$ ) multiplied by mass $m$ . Therefore the work required to move a mass $dm$ is $dW=ahdm.$

Hint 2
For volumes, we can relate the mass ( $dm$ ) to its density ( $\rho$ ) by $\rho dV=dm$ where $dV$ is the small volume occupied by the small mass.

Hint 3
All the water at a given height forms a disk with a radius that depends on the height of the fluid. How can we use this to relate volume and height?

Checking a solution serves two purposes: helping you if, after having used all the hints, you still are stuck on the problem; or if you have solved the problem and would like to check your work.

If you are stuck on a problem: Read the solution slowly and as soon as you feel you could finish the problem on your own, hide it and work on the problem. Come back later to the solution if you are stuck or if you want to check your work.
If you want to check your work: Don't only focus on the answer, problems are mostly marked for the work you do, make sure you understand all the steps that were required to complete the problem and see if you made mistakes or forgot some aspects. Your goal is to check that your mental process was correct, not only the result.

Solution
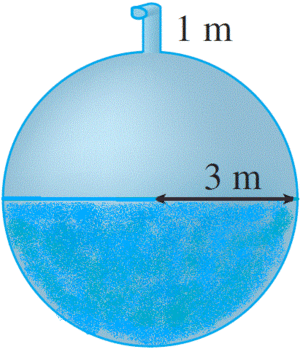
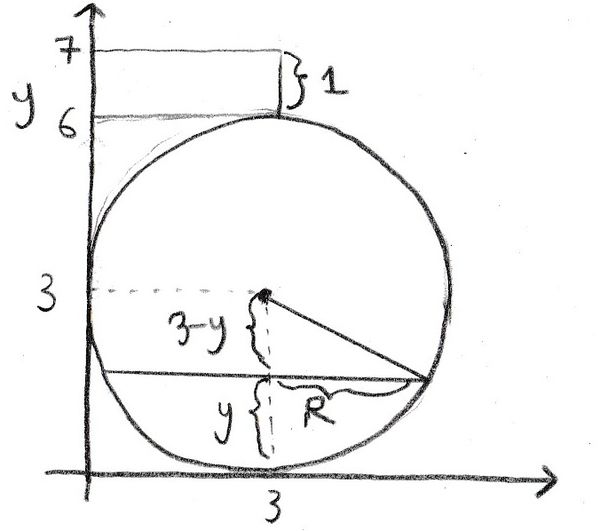
Before beginning, note that all of the units are compatible with each other (i.e. metres go with cubic metres) and so we can just use numbers. However, in general, make sure you convert to the correct units before solving the problem. First, if we describe the given situation on the graph, we get . The formula for work is ${\begin{aligned}dW=ahdm\end{aligned}}$ with $dm$ a small mass, $h$ the distance from the mass to where we want the water to go, and $a$ , the acceleration to move the mass. Note that the mass of water and the distance from the water surface to the top of the tank are changed, as we pump the water. Thus, let’s introduce a variable $y$ which represents the depth of the water as measured from the bottom of the tank. (See the above picture). The goal then is to come up with an integral involving this variable (i.e. we want acceleration, distance out of the tank, and mass to be written in terms of $y$ ). First, since the only force on the water we have to overcome is from gravity, the acceleration is constant and given by Earth's gravitational field, $a=g=9.8$ . Next, since we're dealing with a fluid, it is often easier to use the density instead of mass directly, i.e. we can relate $dm$ to the volume it takes up $dV$ with the fluid density $\rho$ , via, $dm=\rho dV.$ At a fixed depth, $y$ , it will be the same amount of work to remove a single drop as it will the whole disk of water at this depth. Denote the radius of the circle at depth $y$ by $R=R(y)$ as in the picture. Then, $R$ satisfies ${\begin{aligned}R(y)={\sqrt {3^{2}-(3-y)^{2}}}.\end{aligned}}$ Therefore, the amount of volume we need to remove at depth $y$ is the small cylinder made from the disk of radius $R(y)$ with a small amount of depth, $dy$ , $dV=\pi R(y)^{2}dy=\pi (9-(3-y)^{2})dy=\pi (6y-y^{2})dy.$ Finally, to setup the work, we need the height it takes to get the water out of the tank as a function of $y$ . This last step is important and often the most overlooked since at first glance it may seem like this height is the same as $y$ itself since they both measure distance. However, remember that $y$ is the depth measured from the bottom of the tank but we need to get the water out of the top of the tank. From the picture we see that the distance from the top of the tank to the bottom of the tank is 7. As the depth increases the distance to the top of the tank decreases as $h=7-y.$ Plugging everything together and using from the problem that $\rho =1000$ , ${\begin{aligned}dW=(9.8)(7-y)\cdot 1000\pi (6y-y^{2})dy.\end{aligned}}$ For the work required to pump all of the water in the tank, the range for $y$ is from 0 to 3, which implies ${\begin{aligned}W&=\int _{0}^{3}(9.8)(7-y)\cdot 1000\pi (6y-y^{2})dy=9800\pi \int (7-y)(6y-y^{2})dy\\&=9800\pi \int _{0}^{3}y^{3}-13y^{2}+42ydy=9800\pi \left[{\frac {y^{4}}{4}}-13{\frac {y^{3}}{3}}+21y^{2}\right]_{y=0}^{y=3}\\&=9800\pi \left({\frac {3^{4}}{4}}-13{\frac {3^{3}}{3}}+21\cdot 3^{2}\right).\\&=904050\pi \end{aligned}}$