Waves

This article is part of the PhysicsHelp Tutoring Wiki

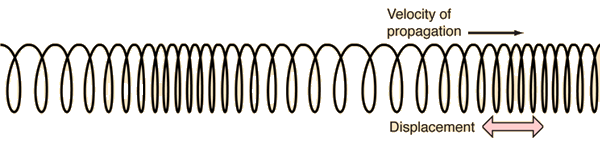
Wave Motion Along a String

This image is a great illustration of how a wave propogates along a string. Try to imagine a string as a chain of pearls or atoms or a chain of some other sort of small segments. Each segment's position is determined by its interactions with its neighbours. When you proffessor wips the end of a string s/he is giving the final segment in the chain enrgy that will be translated to the next segment and so on.

Standing Waves

This image is a great illustration of how a wave will invert when it reaches a fixed point OR MORE DENSE STRING. The force exerted on the more dense segment will not be enough to affect it. Think of it as a tug of war between the two segments, one heavy and one light. When the light segment applies a force to the heavy segment (a heavy rope or a fixed object) the heavy segment is not effected. The force that the light segment applies actually affects itself, so much that the wave is translated in the opposite direction. Try sitting on a rolling chair and attempt to move the table, a force to the left will move you the right.

This image shows the first six specific frequencies that result in minimal energy dissipation; resonance. Each time the sting is pulled through a cycle the motion of the force driving the string, and the harmonic motion of the string itself, act in harmony. The string has a specific frequency that it will oscillate at if displaced from equilibrium and allowed to oscillate back to equilibrium.

You can create a standing wave on a string, fixed at one end, by moving your hand up and down at a one of the resonance frequencies of the string. The fundamental frequency is one that allows the wave reflected off of the wall to match the motion of your hand when it returns to the end of the rope in your hand. The speed of the wave you deliver to the rope is a function of the rope’s physical properties, nothing else. What you need to do is deliver a continuous sinusoidal wave to the rope with a frequency that will produce a wavelength that is a multiple of the length of the rope.

Musical Wind Instruments

This image illustrates how musical wind instruments can create notes from the pressure waves (sound) in air. Keep in mind that the illustration is for pressure waves not waves on a rope. Remember that pressure waves are longitudinal not transverse, this is important when visualizing this physical phenomenon. A pressure wave would be better illustrated by a slinky than a rope.

Closed-closed pipes will exhibit similar patterns as the open-open pipes, however, the nodes will always occur at the edges (instead of the maximum amplitudes of the waves).

Transverse Waves

At each anti-node the pressure is oscillating at maximum amplitude and at the fundamental frequency. The picture may look like the pressure is continuously at maximum value but you need to remember it is oscillating. When a pressure wave encounters a wall or the end of a pipe it is reflected at 180 degrees. For example, if the pressure wave is decreasing from a maximum value to zero at the wall it will be increasing to a maximum once it reflects from the wall.

For a pipe that is open at both ends, the wave is also reflected once it travels the length of the pipe.

Beats

Beats is a special phenomenon used by musicians to tune their instruments. It occurs when two waves travel through the same medium with slightly different frequencies. The image above shows two waves that have almost the same wavelength and therefore almost the same frequency (that is if they are traveling through the same medium). When the two waves are superimposed, as they would be if they were two sound waves traveling through air, the resulting wave is the third wave in the illustration. You will notice that where the waves are in phase the resultant wave will have its maximum amplitude and where the two waves are 90 degrees out of phase the resultant wave has amplitude of zero. What you will hear if the resultant wave was passing by your ear would be a sound like a note on a piano, but the intensity of the note will be fluctuating (maybe a couple of times per second) from high to low while the frequency of the sound will be close to the average of the two frequencies. The frequency of the beating phenomenon is difference in frequencies between the two initial waves.

• Back to PhysicsHelp