Course:PHYS341/Archive/2016wTerm2/AcousticsofReedInstruments
Acoustics of Single Reed Instruments
Reed instruments are a subset of the broader woodwind category of instrumental classification. Reed instruments are primarily characterized by the cane, or synthetic, reed that is set to vibrate in order to produce a musical sound.
When an initial stream of air is shot into the mouthpiece, the production of sound is guided by Hermann von Helmholtz' "inward-striking reed" principle as well as Bernoulli's principle in fluid dynamics.[1]Cite error: Invalid <ref> tag; invalid names, e.g. too many[2]
Component Breakdown of Single Reed Instruments
From stringed to wind, all instruments produce sounds following a similar sequence:
A resonator is forced to oscillate by a particular means of excitation and the resulting vibrations are transferred to the surrounding air in a suitable manner[3]
Each instrument has three central components: an excitation mechanism, a resonator, and a radiation element. In reed instruments, the components are made up of the following:
- Excitation Mechanism: Combination of the performer, the reed and the mouthpiece
- Resonator: Air column
- Radiation element: Tone lattice and air-column's open end [3]
For reed instruments, the excitation begins when the performer blows into the mouthpiece which allows the energy to begin, and then maintain, resonating sound waves in the air column.
Standing Wave in a Wind Instrument
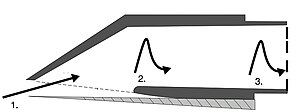
A standing wave is necessary for a musical instrument to produce a musical sound. For string instruments, the standing wave is relatively easy to envision. The motion of a string hitting the bridge is a example of this phenomenon. For wind instruments, visualizing a standing wave is more difficult because we cannot see a physical representation as we would on a string instrument. In wind instruments, the initial wave travels through the resonator (the body of the instrument) and is reflected back towards the excitation mechanism. This is caused by the impedance change. As the wave reaches the end of the instrument, the lower impedance of a free and unrestricted environment, as opposed to the narrow pipes, acts as the bridge of a string instrument, reflecting the wave back through the original medium.[4] Figures 1, 2, and 3 illustrate this process. Note, because the instrument itself is just an extension of the air column, the mouthpiece is sufficient to demonstrate this process.
Reed Vibration and Bernoulli's principle
At rest, there is a naturally occurring aperture between the reed and the baffle. The movement of the reed is determined by the pressure difference between the performer's oral cavity (poc) and the pressure in the mouthpiece (pr).[3][5][1]
By blowing into the mouthpiece, a musician's mouth puts an initial volume of pressure on the reed, pushing it towards the baffle. According to Bernoulli's principle of fluid dynamics, "if it is caused to move across a thin flat surface, the pressure drops below the static air pressure."[2]
Simply put, the jet of air produced by a musician creates a low-pressure stream. Because of the flexibility of the reed, when the pressure within the mouthpiece cavity is negative which, according to Bernoulli's principle, is when the fluid velocity is greatest (i.e., the performer's air input), the cane will "bend" until it eventually closes the aperture entirely (see Figure 4).
When sound waves are reflected back into the mouthpiece, the standing wave creates an equilibrium which reduces the velocity and increases the pressure. In turn, this pushes the reed away from the baffle. The performer can subsequently re-introduce a jet of air into the instrument to restart the cycle (see Figure 5). The continued vibration of the reed, and consequently the standing wave, is thus a result of both the blowing pressure emanating from the player's vocal tract and the Bernoulli principle.[6]
Helmholtz' Inward-Striking Reed
To distinguish between various reed instruments, Helmholtz characterized certain instruments as "inward-striking" and others as "outward-striking."[7][8]

The primary difference between the two reed-types relates to the effects of the pressure differences. For inward-striking, an increase in pressure closes the reed; the opposite is true for outward-striking (see Figure 6).[7][8]
Though the reed's typical natural frequency is assumed to be 2000-3000 Hz, the air column will resonate at much lower frequencies.[2] For inward-striking reeds, the reed itself has only a small influence on the playing frequency.[8]
How to use a reed mouthpiece
The resonance frequency of the vibrating reed can be changed multiple ways. For example:
- Lip Placement: If the performer moves his or her lips away from the tip of the reed, the damping of the reed will be lessened.[3]
- Lip Pressure: The greater the initial pressure on the reed and mouthpiece, the closer the reed is to the baffle. This reduces the effective vibrations of the reed by increasing its stiffness.[3]
Both of these examples would effectively increase the resonance frequency.
- If the performer's lips are placed on the edge of the mouthpiece (at the tip of the reed), there will be no sound produced as the reed will have no room to vibrate.[3]
Mouthpiece Geometry
While the reed acts as a pressure controlled air valve, the cavity within the reed mouthpiece has significant control over the instrument's tone. The mouthpiece is neither cylindrical nor conical. As a result, the sound waves are propagated through the mouthpiece in complex ways.
The quality of tone uses highly subjective terms. Many attempts to establish a relationship between "geometric proportions [of the mouthpiece] and acoustical behaviour have failed"[3]. However, there is a consensus surrounding the timbre of reed instruments based on the dimensions of the mouthpiece. A short and wide mouthpiece is often associated with "dark" tones, while a longer and narrower one is associated with "bright" tones.[3]
See also
References
- ↑ 1.0 1.1 Bilbao, Stefan. "Direct Simulation of Reed Wind Instruments." Computer Music Journal, Vol. 33, No. 4 (Winter 2009), p.44-45.
- ↑ 2.0 2.1 2.2 White, Harvey E. and Donald H. White. Physics and Music: The Science of Musical Sound. Mineola, NY: Dover Publications, Inc. 2014. p.236-237.
- ↑ 3.0 3.1 3.2 3.3 3.4 3.5 3.6 3.7 Scavone, Gary Paul. An Acoustic Analysis of Single-Reed Woodwind Instruments with an Emphasis on Design and Performance issues and Digital Waveguide Modeling Techniques. Stanford University PhD Dissertation. Palo Alto, CA: Stanford University.
- ↑ Lapp, David R. The Physics of Music and Musical Instruments. Wright Centre for Innovative Science Education, Tufts University. Medford, MA: Tufts University, p. 72.
- ↑ Adachi, Seiji. "Principles of sound production in wind instruments." Acoustic Science and Technology. 25, 6 (2004).
- ↑ Benade, Arthur H. "The Physics of Woodwinds." Scientific American. October, 1960. p. 144
- ↑ 7.0 7.1 Fletcher, Neville H. and Thomas D. Rossing. "Sound Generation by Reed and Lip Vibrations." Chapter in The Physics of Musical Instruments. NY: Springer. 1991. p. 401-428.
- ↑ 8.0 8.1 8.2 8.3 Chaigne, Antoine and Jean Kergomard. Acoustics of Musical Instruments. NY: Springer. 2013. p. 479; 537-538.