Course:MATH102/Question Challenge/2008 December Q9

	Questions? Click here to add or read comments for this problem
Please rate how easy you found this problem: {{#w4grb_rate:}} Hard                                              Easy

Question

Graduate student Ryan Lukeman studied the behaviour of duck flocks swimming near Canada Place in Vancouver, BC. This figure from his PhD thesis shows his photography set-up. Here $H=10$ meters is the height from sea level up to his camera aperture at the observation point,$D=2$ meters is the width of a pier (a stationary platform whose size is fixed), and $x$ is the distance from the pier to the leading duck in the flock (in meters). $\alpha$ is a visual angle subtended at the camera, as shown.

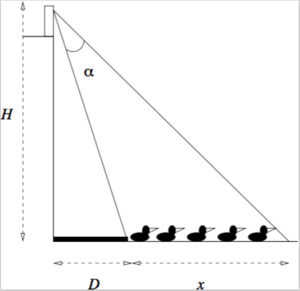
An observer at height H photographing ducks.

If the visual angle is increasing at the rate of 1/100 radians per second, at what rate is the distance $x$ changing at the instant that $x=3$ meters?

${\displaystyle }$

Hints

[show]Hint 1
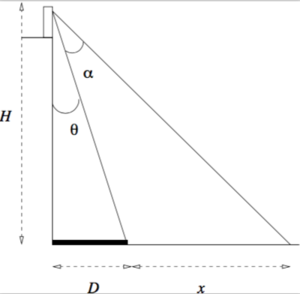Consider the angles $\theta +\alpha$ and $\alpha$ and write down trigonometric functions relating these angles to the distances in the diagram.

Solutions

[show]Solution

Consider the angles in the diagram, and note the definition of the angle $\theta$. Consider the angles $\theta +\alpha$ and $\alpha$ and write down trigonometric functions relating these angles to the distances in the diagram. Then $\tan \theta ={\frac {D}{H}}={\frac {2}{10}}={\frac {1}{5}}$. $\tan(\theta +\alpha )={\frac {D+x}{10}}={\frac {2+x}{10}}$. $\arctan \left({\frac {2+x}{10}}\right)=\theta +\alpha$ $\alpha =\arctan \left({\frac {2+x}{10}}\right)-\theta$ We are told that the visual angle $\alpha$ is increasing at the date of 1/100 radians per sec. This means that ${\frac {d\alpha }{dt}}={\frac {1}{100}}$ Taking the first derivative: ${\frac {d\alpha }{dt}}={\frac {1}{1+((2+x)/10)^{2}}}\cdot {\frac {1}{10}}\cdot {\frac {dx}{dt}}$ ${\frac {1}{100}}={\frac {1}{1+1/4}}\cdot {\frac {1}{10}}\cdot {\frac {dx}{dt}}$ ${\frac {1}{100}}={\frac {4}{5}}\cdot {\frac {1}{10}}\cdot {\frac {dx}{dt}}$ ${\frac {dx}{dt}}={\frac {50}{400}}={\frac {1}{8}}=0.125$ meters per second. If the visual angle is increasing at $1/100$ radians per second, then at the instant $x=3$meters, ${\frac {dx}{dt}}=1/8=0.125$ meters per second.