Course:MATH102/Question Challenge/2000 December Q9

	Questions? Click here to add or read comments for this problem
Please rate how easy you found this problem: {{#w4grb_rate:}} Hard                                              Easy

Question

A wheel of radius 1 meter rolls on a flat surface without slipping. The wheel moves from left to right, rotating clockwise at a constant rate of 2 revolutions per second.

Stuck to the rim of the wheel is a piece of gum, (labeled $G$); as the wheel rolls along, the gum follows a path shown by the wide arc (called a "cycloid curve) in the diagram.

The $(x,y)$ coordinates of the gum ($G$) are related to the wheel's angle of rotation $\theta$ by the formulae

$x=\theta -\sin \theta ,$

$y=1-\cos \theta ,$

where $0\leq \theta \leq 2\pi$.

How fast is the gum moving horizontally at the instant that it reaches its highest point? How fast is it moving vertically at that same instant?

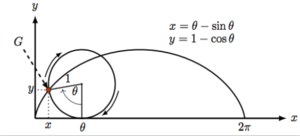
The cycloid curve is formed by a point on the rim of a circle as it rolls along the x axis. Figure made by Sophie Burrill for LE Keshet OpenBook.

Hints

[show]Hint 1
For what angle θ is the gum is at its highest point?

[show]Hint 2
Find the related rate equation through unit analysis. You want to end up with m/s.

[show]Hint 3
One of the derivatives in the related rate equation involves "2 revolutions per second". Remember that 1 revolution is $2\pi$ radians.

Solutions

[show]Solution

We are given that $r=1=$ is the radius of the wheel ${\frac {d\theta }{dt}}=2\pi \cdot 2=4\pi =$ constant $x=\theta -\sin \theta$ $y=1-\cos \theta$ When gum is at its highest point, the circle has gone through 1/2 a full rotation, so the angle $\theta$ (relative to the starting position) is $\theta =\pi$ radians. (Comment: another way of concluding this is to note that $2=1-\cos \theta$ so $\cos \theta =1-2$ $\cos \theta =-1$ so hence $\theta =\pi$ radians. Next we obtain ${\frac {dx}{dt}}$ by differentiating: ${\frac {dx}{dt}}={\frac {d\theta }{dt}}-\left(\cos \theta \cdot {\frac {d\theta }{dt}}\right)$ ${\frac {dx}{dt}}=4\pi -\cos \pi \cdot 4\pi$ ${\frac {dx}{dt}}=4\pi +4\pi$ ${\frac {dx}{dt}}=8\pi$ Finally let's solve for ${\frac {dy}{dt}}$ by differentiating: ${\frac {dy}{dt}}=-\left(-sin\theta \cdot {\frac {d\theta }{dt}}\right)$ ${\frac {dy}{dt}}=\sin \pi \cdot 4\pi$ ${\frac {dy}{dt}}=0$