Stellar Atmospheres

Reading Assignment

Introduction

Inside the star you only needed the state variables: $P,T,\rho$ as a function of position and you tacitly assumed that everything is in thermodynamic equilibrium locally.

Stellar spectra would be really uninteresting if this worked all of the way out. In the atmosphere the mean-free path for a photon is longer or similar to the distance to open space. The optical depth to infinity is small. Therefore, we need to keep track of the photon distribution in energy, direction and polarization too. In principle, the matter need not be in thermodynamic equilibrium either.

Calculate the radiation field given the atmosphere structure.
Correct the atmosphere structure so energy is conserved throughout.
Repeat until converged.

Breakdown of strict thermodynamic equilibrium
Assumption of LTE: matter in TE, but photons are not.
Continuum is assumed to determine the structure and spectral lines form on top.
Atmosphere is thin; little mass (but think about red giants)

${\frac {dP}{dr}}=-{\frac {GM(r)\rho }{r^{2}}}=-g\rho$

and

${\frac {dF}{dr}}=0$

so $F=\mathrm {constant} =\sigma T_{e}^{4}$ . Two important parameters:

$g={\frac {GM}{R^{2}}}$ and
$T_{e}^{4}={\frac {L}{4\pi R^{2}}}$ .

To follow the photon distribution you need to use the equations of radiative transfer.

Schwarzschild-Milne Integral Equations

Using this let's develop the Schwarzschild-Milne Integral Equations

$S_{\nu }=\epsilon _{\nu }B_{\nu }+(1-\epsilon _{\nu })J_{\nu }$

where

$\epsilon _{\nu }={\frac {\alpha _{\nu }}{\alpha _{\nu }+\sigma _{\nu }}}.$

N.B. $\alpha _{\nu }$ deals with true absorption and $\sigma _{\nu }$ deals with scattering.

Let's split the radiation into outgoing and ingoing

$J_{\nu }={\frac {1}{2}}\int _{0}^{1}I(+\mu ',\tau _{n}u)d\mu '+{\frac {1}{2}}\int _{0}^{1}I(-\mu ',\tau _{n}u)d(-\mu ')$

so

$J_{\nu }={\frac {1}{2}}\int _{0}^{1}\left[\int _{\infty }^{\tau _{\nu }}S(t)e^{(\tau _{n}u-t)/\mu '}{\frac {dt}{\mu '}}\right]d\mu '+{\frac {1}{2}}\int _{0}^{1}\left[\int _{0}^{\tau _{\nu }}S(t)e^{(\tau _{n}u-t)/\mu '}{\frac {dt}{\mu '}}\right]d\mu '$

and

$J_{\nu }={\frac {1}{2}}\int _{0}^{\infty }S(t)\left[\int _{0}^{1}e^{|\tau _{\nu }-t|/\mu '}{\frac {d\mu '}{\mu '}}\right]dt.$

If we define the exponential integral

$E_{n}(z)=\int _{1}^{\infty }{\frac {e^{-zt}}{t^{n}}}dt=\int _{0}^{1}e^{-z/y}y^{n-2}dy,$

we have

$J_{\nu }(\tau _{n}u)={\frac {1}{2}}\int _{0}^{\infty }S(t)E_{1}|\tau _{\nu }-t|dt$

and

$S_{\nu }(\tau _{\nu })=\epsilon _{\nu }B_{\nu }(T(\tau _{\nu }))+(1-\epsilon _{\nu }){\frac {1}{2}}\int _{0}^{\infty }S(t)E_{1}|\tau _{\nu }-t|dt$

Limb Darkening

$I(\mu ,0)=\int _{0}^{\infty }S(t)e^{-t/\mu }{\frac {dt}{\mu }}={\frac {1}{\mu }}{\mathcal {L}}_{1/\mu }\left[S(t)\right].$

so if $S(t)=at+b$ , $I(\mu ,0)=a\mu +b$ .

Spectral Lines

Spectral lines form one of the most powerful diagnostics of a star's atmosphere.

The Voigt profile is a line profile resulting from the convolution of two broadening mechanisms, one of which alone would produce a Gaussian profile (usually, as a result of the Doppler broadening), and the other would produce a Lorentzian profile. Voigt profiles are common in many branches of spectroscopy. Due to the computational expense of the convolution operation, the Voigt profile is often approximated using a pseudo-Voigt profile.

Without loss of generality, we can consider only centered profiles, which peak at zero. The Voigt profile is then a convolution of a Lorentz profile and a Gaussian profile:

V(x;\sigma ,\gamma )=\int _{-\infty }^{\infty }G(x';\sigma )L(x-x';\gamma )\,dx',

where x is the shift from the line center, $G(x;\sigma )$ is the centered Gaussian profile:

$G(x;\sigma )\equiv {\frac {e^{-x^{2}/(2\sigma ^{2})}}{\sigma {\sqrt {2\pi }}}},$

and $L(x;\gamma )$ is the centered Lorentzian profile:

L(x;\gamma )\equiv {\frac {\gamma }{\pi (x^{2}+\gamma ^{2})}}.

The Gaussian results from the temperature of the gas and the Lorentzian results from the collision rate in the gas, so a spectral line provides a estimate of both the temperature and density of the atmosphere. Furthermore, if the star is also rotating, this will also broaden the lines further.

To account for the strength of a spectral line, there are several conventions.

A diagram indicating the equivalent width corresponding to the absorption line, which is shown in red.

The residual flux and residual intensity are defined with respect to the continuum surrounding the line,

$f_{\nu }(\mu )\equiv {\frac {I_{\nu }(\mu ,0)}{I_{c}(\mu ,0)}}\equiv \mathrm {residual~intensity}$

and

$r_{\nu }\equiv {\frac {F_{\nu }(0)}{F(0)}}\equiv \mathrm {residual~flux} .$

The equivalent width of a spectral line is a measure of the area of the line on a plot of intensity versus wavelength. It is found by forming a rectangle with a height equal to that of continuum emission, and finding the width such that the area of the rectangle is equal to the area in the spectral line.

Formally, the equivalent width is given by the equation

$W_{\lambda }=\int (1-F_{\lambda }/F_{0})d\lambda$ .

Here, $F_{0}$ represents the continuum intensity on either side of the absorption or emission feature, while $F_{\lambda }$ represents the intensity across the entire wavelength range of interest. Then $W_{\lambda }$ represents the width of a hypothetical line which drops to an intensity of zero and has the "same integrated flux deficit from the continuum as the true one." This equation can be applied to either emission or absorption, but when applied to emission, the value of $W_{\lambda }$ is negative, and so the absolute value is used.

Schuster-Schwarzschild Model

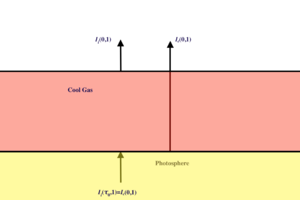
Sketch of Schuster-Schwarzschild model for line formation

This is based on the Eddington approximation and we assume that the lines are actually scattering lines. The gas immediately radiates the energy in a different direction, so we have

$\mu {\frac {dI_{v}}{d\tau _{\nu }}}=I_{\nu }-J_{\nu }$

and

$d\tau _{\nu }=-\sigma _{\nu }dx$

Because the scatter is isotropic the flux is conserved. If we average the radiative transfer equation over direction

${\frac {dH_{\nu }}{d\tau _{\nu }}}=J_{\nu }-J_{\nu }=0$

and because $H_{\nu }\propto F_{\nu }$ , the flux is conserved. Let's take $I_{\nu }=a_{\nu }(z)+\mu b_{\nu }(z)$ so

$J_{\nu }=a_{\nu },H_{\nu }={\frac {b_{\nu }}{3}},K_{\nu }={\frac {a_{\nu }}{3}},$

${\frac {dK_{\nu }}{d\tau _{\nu }}}={\frac {1}{3}}{\frac {dJ_{\nu }}{d\tau _{\nu }}}=H_{\nu }$

and

${\frac {dJ_{\nu }}{d\tau _{\nu }}}=b_{\nu }.$

We can solve these equations

$I_{\nu }^{l}(\tau ,\nu )=b_{\nu }(\tau _{\nu }-\tau _{0})+b_{\nu }\mu +Q$

at $\tau _{\nu }=\tau _{0}$ and $\mu =1$ , $I_{\nu }^{l}(\tau _{0},1)=I_{\nu }^{c}(0,1)$ , so $Q=I_{\nu }^{c}(0,1)-b_{\nu }$ .

Putting it all together yields

$I_{\nu }^{l}(\tau ,\mu )=b_{\nu }(\tau _{\nu }-\tau _{0})+b_{\nu }\mu +I_{\nu }^{c}(0,1)-b_{\nu }.$

On the outer surface ( $\tau _{\nu }=0$ ) there is no incoming intensity so

$I_{\nu }^{l}(0,-1)=0=b_{\nu }(-\tau _{0})-b_{\nu }+I_{\nu }^{c}(0,1)-b_{\nu },$

$b_{\nu }(2+\tau _{0})=I_{\nu }^{c}(0,1)$

and

$I_{\nu }^{l}(\tau ,\mu )=I_{\nu }^{c}(0,1)\left[{\frac {\tau _{\nu }+(\mu +1)}{2+\tau _{0}}}\right].$

Therefore, the residual intensity and flux are given by

$f_{\nu }={\frac {\mu +1}{\tau _{0}+2}},r_{\nu }={\frac {2}{\tau _{0}+2}}$

Assignment

Assume the following:

The Rosseland mean opacity is related to the density and temperature of the gas through a power-law relationship,

$\kappa _{R}=\kappa _{0}\rho ^{\alpha }T^{\beta };$

The pressure of the gas is given by the ideal gas law;
The gas is in hydrostatic equilibrium so $p=g\Sigma$ where $g$ is the surface gravity; and
The gas is in radiative equilibrium with the radiation field so the flux is constant with respect to $z$ or $\Sigma$ .

Calculate the temperature of the gas as a function of $\Sigma$ for a general opacity law.

Now use the specific opacity law

$\kappa _{R}=3.68\times 10^{22}g_{ff}(1-Z)(1+X){\frac {\rho }{1~\mathrm {g~cm} ^{3}}}\left({\frac {T}{1\mathrm {K} }}\right)^{-7/2}\mathrm {cm} ^{2}\mathrm {g} ^{-1}$

and take the value of the gravitational acceleration and effective temperature appropriate for the Sun to get an estimate for the run of temperature with column density for the Sun.

Now let's try to estimate the continuum spectrum under the following assumptions

The opacity is a power-law function of wavelength: $\kappa _{\nu }\propto \nu ^{-\delta }$ .
The emission from the atmosphere is thermal.
The radiation is coupled to the atmosphere at optical depths $\tau _{\nu }$ greater than unity and completely decoupled at shallower depths where $d\tau _{\nu }=\kappa _{\nu }d\Sigma /(\cos \theta )$ .

What does the spectrum ( $I_{\nu }$ ) look like for $\delta =0$ as a function of $\theta$ ? What about $\delta =2$ and $\delta =3$ ?