Science:Math Exam Resources/Courses/MATH104/December 2015/Question 10 (d)/Solution 1

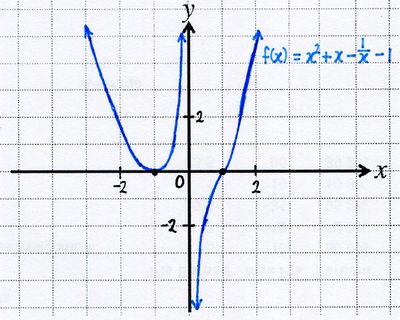
We first find the x-intercepts/roots of $f(x):$

${\begin{aligned}x^{2}+x-{\frac {1}{x}}-1&=0\\x^{3}+x^{2}-1-x&=0\\(x-1)(x+1)^{2}&=0\\x&=\pm 1\end{aligned}}$

(In fact, since $x=-1$ is a root of multiplicity 2, we know that the graph of $f(x)$ just 'touches' the x-axis at $x=-1,$ whereas at the simple (i.e., multiplicity 1) root $x=1$ it crosses the x-axis.)

We plot these on our grid:

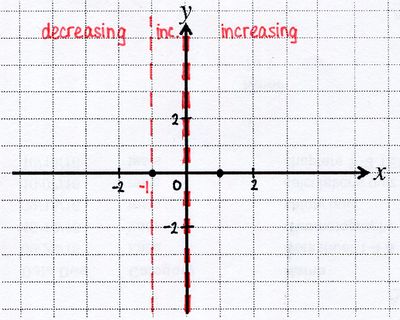
From 10 (b), we know that $f(x)$ is increasing on $[-1,\infty )\setminus \{0\}$ and decreasing on $(-\infty ,-1].$

From 10 (c), we know that $f(x)$ is concave up on $(-\infty ,0)\cup [1,\infty )$ and concave down on $(0,1].$

From 10 (a), we know the behaviour of $f$ around its vertical asymptote $x=0.$ Namely, $\lim \nolimits _{x\to 0^{-}}f(x)=\infty$ and $\lim \nolimits _{x\to 0^{+}}f(x)=-\infty .$ Combining all the above information, we obtain the following sketch:

Note: It is helpful to evaluate $f$ at integer values (which should be relatively easy to do), e.g., $x=-3,-2,2$ to guide your sketch.

Finally, the complete sketch: