Documentation:FIB book/problems/Nij Whiplash

A study was conducted to investigate the effect of seat-back stiffness on the risk of whiplash injuries in rear-end collisions .The Hybrid III mid-sized male dummy was used to perform the tests.

Question 1

Researchers used the $N_{ij}$ criteria to estimate the risk of injury which is calculated as follows:

$N_{ij}={\frac {F_{z}}{F_{zc}}}+{\frac {M_{y}}{M_{yc}}}$

Where $F_{z}$ and $M_{y}$ are axial force (tension or compression) and moment (flexion or extension), respectively, and $F_{zc}$ and $M_{yc}$ are their respective critical values.

Four types of neck injury criteria are (Figure 1):

$N_{TF}$: Tension-Flexion

$N_{TE}$: Tension-Extension

$N_{CF}$: Compression-Flexion

$N_{CE}$: Compression-Extension

Among four types of neck injury criteria, which one you find the best estimator for whiplash injuries? Why? (Hint: Think about the mechanism of whiplash injury and the fact that most whiplash injury protection devices such as headrests try to prevent the head from backward movement)

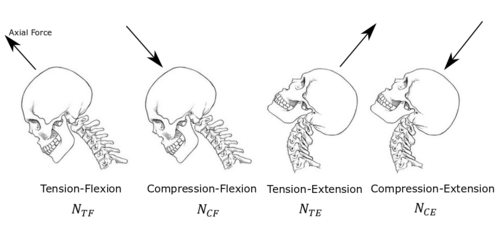
Figure 1- Different flavors of $N_{ij}$

[show]SOLUTION (expand to show)

It is postulated that whiplash injuries occur due to hyper-extension and hyper-tension of the neck. During the final phase of the whiplash injury mechanism where the head is moving rearward, both ends of the neck are in extension and at the same time, the whole neck system experiences tensile loads. Under this pattern of loading, a tension-extension injury is more likely to happen compared to other scenarios. This is why in most whiplash injury studies $N_{TE}$is calculated, and most protection devices try to support head from the back.

Question 2

You are given the data for axial force and flexion-extension moment of neck load cells at “rigid” and “not rigid” (175 Nm/deg) recliner stiffnesses. Use this data to calculate the maximum value of the criterion from the previous question for two given recliner stiffnesses and explain the procedure. (You can use the page SES-5 of this file. This page is on the 7th page of the PDF)

Hint:

Positive moment represents flexion and positive force represents tension

Download the data here

[show]SOLUTION (expand to show)

To calculate the criterion value, firstly we should find the critical values for extension and tension loads. Based on the table SES-1 in the provided file, the critical values for mid-sized male HIII dummy are as follows (Table 1): Tension: 6806 N Extension: 135 Nm Table 1- Critical intercepts used for calculating $N_{TE}$ After that, we should find in which range of the data extension and tension occur at the same time, which means searching for a range where the moment is negative and the force is positive. This happens in the range 123:210 for the rigid recliner; and in the ranges 154:237, 278:297, 318:355 for the non-rigid recliner. Using the formula, we should calculate the criterion values for all the ranges and find the maximum value. It is important to use the absolute values of the moment. Calculations lead to the following criterion values for all the ranges: Recliner Range $N_{TE}$value Rigid 123:210 1.2477 Flexible 154:237 0.8905 Flexible 278:297 0.0291 Flexible 318:355 0.1038 Therefore, $N_{TE}$for the rigid recliner is 1.2477 versus 0.8905 for the flexible one.

Question 3

Compare the two recliners based on the calculated criterion.

[show]SOLUTION (expand to show)
As injury criteria are designed to estimate the risk of injury and $N_{TE}$ is higher in a rigid recliner, a flexible recliner results in a lower risk of injury compared to a rigid one.