Course:MATH110/Archive/2010-2011/003/Teams/Valais/Homework 12

Team Valais: Homework 12

Problem

Team Problem

Starting with the function $P(t)={\frac {1}{1+e^{-t}}}$ Your goal is to modify the function so that we can use it to model a real-life problem. We want to be able to control the following things: Change the height of the horizontal asymptote on the right, we'll denote it by $K$. Change the $y$-intercept to any number between $0$ and $K$ BONUS (just the point below, not what comes after) Change the slope of the curved part. Find a way so that the slope can go from very close to zero to almost vertical. (If you graph it, it should be quite clear). Once you've played with the function enough, try to find an application of the graph to model something. It can be anything which starts at a value and then goes to another one (think for a population, it goes from 0 to it's carrying capacity). Explain what you are modelling and how you decide to attribute a numerical value to each of the 2 or 3 parameters that you researched just above. Then use the model to make a prediction. For example, if your model is suppose to describe a population for which you have its initial population and carrying capacity (potentially its rate of increase if you solved the bonus part), then use that data to make a prediction for the population in 20 years, or use the model to predict when will the population reach 95% of its carrying capacity). When doing this last part, explain well where you're taking your data from (real data or imagined data), what it is that you're modelling and how you are doing the math to answer a predictive question.

Solution

In order to solve this problem we first have to look at the general shape of the graph. When graphing the function it will look very similar to the following graph:

For the first problem to solve we have to put the k-value after the whole equation in order to move the horizontal asymptote to any direction. The formula would look like :

Now if we change this number to k=2 our horizontal asymptote would be at k+1 (3):

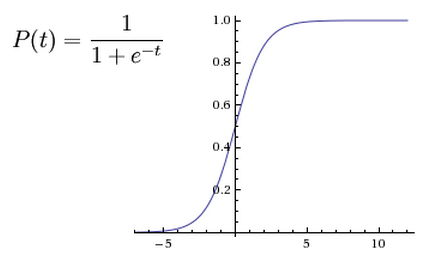
So as we can see our horizontal asymptote is at 3 when k=2.