Course:PHYS350/Phase Space

What is it?

The structure of Hamilton's equations encourage us to think of the motion of particles taking place in phase space. Phase space consists of both the coordinates and the momenta. The location of a particle in phase space is $(q_{1},q_{2},\ldots q_{n},p_{1},p_{2},\ldots p_{n})$. The velocity of a particle in phase space is determined by Hamilton's equations.

${\dot {q}}_{i}={\frac {\partial H}{\partial p_{i}}},{\dot {p}}_{i}=F_{i}-{\frac {\partial H}{\partial q_{i}}}$

The trajectory of a particle through the phase space follows the flow lines given by Hamilton's equations.

If we assume that neither the generalised forces nor the Hamiltonian are explicit functions of time, then the velocity of a particle in phase space depends only on its position in phase space. In this case, the trajectories of particles cannot intersect.

An example will make this clear.

A Pendulum in Phase Space

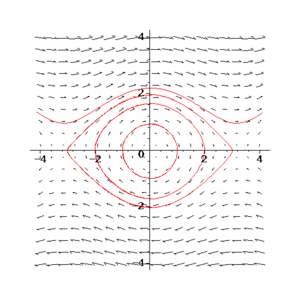
Phase-space trajecories for a pendulum

We calculated Hamilton's equations for a pendulum earlier.

${\dot {p}}_{\theta }=-{\frac {\partial H}{\partial \theta }}=-mgl\sin \theta ,$

${\dot {\theta }}={\frac {\partial H}{\partial p_{\theta }}}={\frac {p_{\theta }}{ml^{2}}}$

For small values of θ, the sine is about equal to the angle itself, so the phase flow swirls around the origin in a clockwise direction. The trajectory of the pendulum is simply an ellipse centered on the origin for small trips through phase space (this is a hallmark of harmonic motion). For larger values of θ, the sine is not nearly equal to the angle itself and the trajectory becomes more distorted and eventually opens up. This latter situation corresponds to the pendulum going round and round rather than back and forth.

Impulses

An impulse is a really big force that operates over a short period of time and then shuts off. The ideal impulse is a force of zero duration that still imparts a finite change in the momentum of a particle. The effect of an impulse on the phase space diagram is simply to force the particle to jump up or down in the direction of changing momentum while the position remains unchanged.

The system then proceeds along a new trajectory.

Sudden Approximation

One can also imagine a sudden and lasting change to the Hamiltonian. Here the location of the system in phase space does not change but the underlying flow field determined by the Hamiltonian does, because we have a new Hamiltonian!

Supernovae and binaries

Let's imagine that we have two stars travelling in a circular orbit. One star suffers a supernova and loses Δ M of material isotropically Will the stars remain bound to each other?

In the sudden approximation both the distance between the stars and their relative velocity (the mass of one of the stars changes in the explosion) will be constant as the mass is lost. The Hamiltonian will change. The pair is still bound if the energy of the system after the mass loss is still negative. Before the explosion we have

$v_{1}^{2}={\frac {GM_{2}}{R}}{\frac {M_{2}}{M_{1}+M_{2}}},v_{2}^{2}={\frac {GM_{1}}{R}}{\frac {M_{1}}{M_{1}+M_{2}}}$

After the explosion the velocities and positions are the same, so we have

$E_{\rm {final}}={\frac {1}{2}}(M_{1}-\Delta M)v_{1}^{2}+{\frac {1}{2}}M_{2}v_{2}^{2}-{\frac {G(M_{1}-\Delta M)M_{2}}{R}}$

Substituting the velocities above gives

$E_{\rm {final}}={\frac {1}{2}}{\frac {M_{2}}{M_{1}+M_{2}}}{\frac {G(M_{1}-\Delta M)M_{2}}{R}}+{\frac {1}{2}}{\frac {M_{1}}{M_{1}+M_{2}}}{\frac {GM_{1}M_{2}}{R}}-{\frac {G(M_{1}-\Delta M)M_{2}}{R}}$

Simplifying this a bit gives

$E_{\rm {final}}=-{\frac {GM_{1}M_{2}}{2R}}\left[1-{\frac {\Delta M}{M_{1}+M_{2}}}\left({\frac {M_{2}}{M_{1}}}+2\right)\right]$

so the system will remain bound if

${\frac {\Delta M}{M_{1}+M_{2}}}<{\frac {M_{1}}{M_{2}+2M_{1}}}$

A little thought will reveal that after the explosion, the stars are located at the minimum separation for their new orbit, and one can calculate the final shape of the orbit as well. Furthermore, the explosion of star #1 might have given this star some momentum -- we can include this as well by using the impulse concept.

The Area Enclosed by the Curve

The area enclosed by a parametric curve is given by $A=\int _{t_{1}}^{t_{2}}p(t){\frac {dq(t)}{dt}}dt$ when the motion is periodic, so all of the coordinates and momenta are equal at t₁ and t₂.

Let's apply this result to the trajectory of a particle through phase space. Furthermore, let's imagine taking the sum of the area of all the curves $\left(q_{i},p_{i}\right)$ when the motion is multidimensional so we have

$A=\oint \sum _{i}p_{i}(t){\frac {dq_{i}(t)}{dt}}dt.$

Let's use the defintion of the Hamiltonian,

$\int _{t_{1}}^{t_{2}}Hdt=\int _{t_{1}}^{t_{2}}\left(\sum _{i}p_{i}{{\dot {q}}_{i}}-L\right)dt=A-\int _{t_{1}}^{t_{2}}Ldt=A-S$

If the Hamiltonian is not an explicit function of time and there are no non-conservative forces we have

$A=\int _{t_{1}}^{t_{2}}(L+E)dt=S+E\left(t_{2}-t_{1}\right)$

so the area of the curve is simply related to the action of the motion.

Adiabatic Changes

Let's imagine a system executes periodic motion and the Hamiltonian is characterized by some parameter λ. It could be the length of a pendulum, the spring constant, the mass of a star etc. Furthermore, let's imagine that λ changes gradually with time, so that

$T{\frac {d\lambda }{dt}}\ll \lambda$

where T is the period of the motion.

If λ were strictly constant, the motion of the system would be closed. In reality, the system doesn't return to exactly the same configuration after a time T. We will neglect this fact and assume that at any time the system executes motion that is characterized by a Hamiltonian that is fixed in time, and average over the motion of the constant Hamiltonian. In general, we have

${\frac {dE}{dt}}={\frac {\partial H}{\partial t}}={\frac {\partial H}{\partial \lambda }}{\frac {d\lambda }{dt}}.$

This expression depends not only on the slowly varying value of λ but also on q and p that vary on a rapid timescale, so we take the average over a period of both sides of the equation to get

${\frac {\overline {dE}}{dt}}={\frac {\overline {\partial H}}{\partial \lambda }}{\frac {d\lambda }{dt}}.$

where

${\frac {\overline {\partial H}}{\partial \lambda }}={\frac {1}{T}}\int _{0}^{T}{\frac {\partial H}{\partial \lambda }}dt$

Let's calculate the period of the motion

$T=\int _{0}^{T}dt=\oint {\frac {dq}{\dot {q}}}=\oint \left({\frac {\partial H}{\partial p}}\right)^{-1}dq$

where the integral from some value of q along the motion until the system returns to the same value of q.

so we have

${\frac {\overline {dE}}{dt}}={\frac {d\lambda }{dt}}\left[\oint \left({\frac {\partial H}{\partial p}}\right)^{-1}dq\right]^{-1}\oint {\frac {\partial H}{\partial \lambda }}\left({\frac {\partial H}{\partial p}}\right)^{-1}dq$

The integrals in the equation above must be taken over the path of the system when the Hamiltonian is constant. When the Hamiltonian is constant we can write the momentum at any time as a function of the position of the system, its energy and the value of λ the Hamiltonian is a function of position, momentum and λ. We have

$p=p(q;E,\lambda ),H(p,q,\lambda )=E,{\frac {\partial H}{\partial \lambda }}+{\frac {\partial H}{\partial p}}{\frac {\partial p}{\partial \lambda }}=0$

so

${\frac {\partial H}{\partial \lambda }}\left({\frac {\partial H}{\partial p}}\right)^{-1}=-{\frac {\partial p}{\partial \lambda }}$

and

${\frac {\overline {dE}}{dt}}=-{\frac {d\lambda }{dt}}\left[\oint {\frac {\partial p}{\partial E}}dq\right]^{-1}\oint {\frac {\partial p}{\partial \lambda }}dq$

where we have replaced $\partial p/\partial H$ with $\partial p/\partial E$. Let's rearrange this expression so that everything is on the right-hand side to get $0={\frac {\overline {dE}}{dt}}\left[\oint {\frac {\partial p}{\partial E}}dq\right]+{\frac {d\lambda }{dt}}\oint {\frac {\partial p}{\partial \lambda }}dq=\oint {\frac {\overline {dE}}{dt}}{\frac {\partial p}{\partial E}}+{\frac {d\lambda }{dt}}{\frac {\partial p}{\partial \lambda }}dq=\oint {\frac {dp}{dt}}dq={\frac {d}{dt}}\oint pdq={\frac {dA}{dt}}$

so the area of that the curve in phase space enclosed is constant for changes in the Hamiltonian that are sufficiently slow. For a harmonic oscillator, this means that the ratio of the energy to the frequency is constant if you change the frequency gradually. You could do this by gradually heating the spring or having the oscillating mass be a box of sand with a small hole.

The Phase-Space Flow

Let's imagine that we start out a whole bunch of systems with various initial conditions $q_{i}(0),p_{i}(0)$. Furthermore, let's imagine that there are so many systems that we can define a function called the phase-space density which tells us how many systems lie in a particular range of phase space.

$dN=f(q_{i},p_{i})\prod dq_{i}dp_{i}$

Because systems cannot appear and disappear we can use the continuity equation to calculate how the phase-space density changes as the systems evolve in time

$0={\frac {\partial f}{\partial t}}+\sum _{i}\left[{\frac {\partial }{\partial q_{i}}}\left({\dot {q}}_{i}f\right)+{\frac {\partial }{\partial p_{i}}}\left({\dot {p}}_{i}f\right)\right]$

Let's substitute Hamilton's Equations into this expression for the velocities through phase space

        $0={\frac {\partial f}{\partial t}}+\sum _{i}\left[{\frac {\partial }{\partial q_{i}}}\left({\frac {\partial H}{\partial p_{i}}}f\right)+{\frac {\partial }{\partial p_{i}}}\left(\left(F_{i}-{\frac {\partial H}{\partial q_{i}}}\right)f\right)\right]$

        $={\frac {\partial f}{\partial t}}+\sum _{i}\left[{\frac {\partial ^{2}H}{\partial q_{i}\partial p_{i}}}f+{\dot {q}}_{i}{\frac {\partial f}{\partial q_{i}}}+{\frac {\partial F_{i}}{\partial p_{i}}}f-{\frac {\partial ^{2}H}{\partial p_{i}\partial q_{i}}}f+{\dot {p}}_{i}{\frac {\partial f}{\partial p_{i}}}\right]$         $={\frac {\partial f}{\partial t}}+\sum _{i}\left[{\dot {q}}_{i}{\frac {\partial f}{\partial q_{i}}}+{\frac {\partial F_{i}}{\partial p_{i}}}f+{\dot {p}}_{i}{\frac {\partial f}{\partial p_{i}}}\right]={\frac {df}{dt}}+\sum _{i}{\frac {\partial F_{i}}{\partial p_{i}}}f$

where we use the defintion of the total derivative

${\frac {df}{dt}}={\frac {\partial f}{\partial t}}+\sum _{i}\left[{\dot {q}}_{i}{\frac {\partial f}{\partial q_{i}}}+{\dot {p}}_{i}{\frac {\partial f}{\partial p_{i}}}\right]$

Liouville's Theorem

We can rearrange the result above to yield

${\frac {df}{dt}}={\frac {\partial f}{\partial t}}+\sum _{i}\left[{\dot {q}}_{i}{\frac {\partial f}{\partial q_{i}}}+{\dot {p}}_{i}{\frac {\partial f}{\partial p_{i}}}\right]=-\sum _{i}{\frac {\partial F_{i}}{\partial p_{i}}}f$

so if there are no dissipative forces we have

${\frac {df}{dt}}=0$

which is Liouville's theorem. We have imagined that the systems do not interact with each other if they do we have a slightly different result

${\frac {df}{dt}}=C-\sum _{i}{\frac {\partial F_{i}}{\partial p_{i}}}f$

where the parameter C accounts for collisions that can scatter pairs of particles from one momentum to another. The collisions effectively make systems disappear from one part of phase space and reappear in another, so the continuity equation that we used earlier is not strictly valid.

Phase-Space Density of a Damped Pendulum

Let's calculate how the phase-space density of a group of non-interacting pendulums changes with time. If there is no friction, the total derivative of the phase-space density vanishes. Let's consider the situation if there is viscous friction. Here we have a power function,

$P=-{\frac {1}{2}}av^{2}=-{\frac {1}{2}}al^{2}{\dot {\theta }}^{2}$

yielding a generalized force of

$F_{\theta }=-al^{2}{\dot {\theta }}=-a{\frac {p}{m}}$

so we have

${\frac {df}{dt}}=C-\sum _{i}{\frac {\partial F_{i}}{\partial p_{i}}}f={\frac {a}{m}}f$

so

$f=f_{0}\exp \left({\frac {at}{m}}\right).$

The phase-space density increases exponentially.